A spacecraft leaves Earth exactly with escape velocity V2 - what trajectory it will have in Solar System?

Question

A textbook solution: if a spacecraft leaves the isolated Earth with escape velocity V2 (=11.2 km/s at Earth's surface) it will have parabolic escape trajectory.

But Earth is in orbit around Sun.

The title question is - what trajectory the spacecraft will have in Solar System?

I suppose the spacecraft will have an orbit around Sun not very different from Earth's orbit (like Spitzer, Kepler and STEREO spacecraft). Flights to Mars and Venus require characteristic energy C3 to be positive, but for parabolic escape C3=0.

-What highest aphelion can be achieved in this case?

-What lowest perihelion can be achieved?

I think trajectory will depend on direction of escape burn. Will it also depend on height of burn too? (Escape velocity changes with distance from gravity center)

-What if escape burn is perpendicular to Earth's orbit plane?

-What are user-friendly tools I could use to evaluate it?

Answer 1

Four questions...

• what trajectory the spacecraft will have in Solar System?

An elliptic orbit. What exact shape depends on the point of departure.

• What highest aphelion can be achieved in this case?

• What lowest perihelion can be achieved?

  Let's take two critical situations: craft tumbling out of the unstable balance of Earth-Sun L1 and L2 respectively.

  Using Lagrange Point Finder, distance of these points from the Sun, and speed at these points will be:

  $$ r_{L1} = 148106319 km \\ r_{L2} = 151099402 km \\ v_{L1} = 29488 m/s \\ v_{L2} = 30084 m/s $$

  $v_{L1}$ is too low for a circular orbit of that altitude, that will be the aphelion of this elliptic orbit. $v_{L2}$ is too high; it's perihelion of that orbit.

  Using Vis Viva equation $ v^2 = GM({2 \over r} - {1 \over a})$ and substituting $M_{sun}$ for $M$, and respective speeds and distances, we obtain the semi-major axis of these orbits.

  $$a = {1 \over {2 \over r} - {v^2\over GM}}$$

  $$ a_{L1} = 1.439×10^8 km \\ a_{L2} = 1.558×10^8 km $$

  Since the major axis (2x semi-major) is equal to the sum of the apses of the ellipse (it's literally the exact same segment) $pe_{L1} = 2a - ap_{L1}$ and $pe_{L1} = 2a - ap_{L2}$

  $pe_{L1} = 1.396×10^8 km$ or 0.933 AU (for 0.99 AU apoapsis)

  $ap_{L2} = 1.606×10^8 km$ or 1.074 AU (for 1.01 AU periapsis)

• What if escape burn is perpendicular to Earth's orbit plane?

  Then you will enter an orbit slightly inclined relative to Earth orbit. Your orbital period won't be all that different, so you're likely to encounter Earth half a year later, and the resulting gravity assist will completely randomize your subsequent orbit. If not, you will have an orbit similar to Earth's but inclined. Neither apoapsis nor periapsis will exceed these of the prior case.

• What are user-friendly tools I could use to evaluate it?

  The aforementioned Lagrange Point Finder to find the precise values, the Vis Viva equation for binding ellipse geometry and orbital mechanics, the Ellipse article for a whole bunch of useful equations relating to ellipse, WolframAlpha for doing the calculations, and Kerbal Space Program to acquire the necessary intuition to know where what happens, like what are the extreme situations where the apses obtain maximum/minimum values.

Answer 2

You are correct - the final orbit will be very similar to Earth's.

A parabolic trajectory necessarily means that relative velocity is equal to 0 at infinite distance or, more helpfully, velocity tends to zero as distance tends to infinity. Also, gravitational acceleration tends to zero.

From this, we can easily approximate that at some large distance from the Earth, an escaping spacecraft (that started from Earth's surface with escape velocity) with have a relative velocity of almost zero. With multi-body systems (such as our Solar System), the Hill Sphere of a body describes this large distance very nicely. For Earth, the Hill Radius is approximately 1.5 million km, or about 0.01 AU - very small compared to Earth's orbital radius. At this Hill Radius, the gravitational acceleration from Earth is $\approx0.00018ms^{-2}$ - also essentially zero.

So what does it mean for a craft to be in approximately the same place as Earth at a given time with approximately the same velocity? They are in the same orbit!

More realistic details

That's the ballpark approximation, but clearly a real world spacecraft in this configuration would not continue co-orbiting with Earth for a long time. The velocity required to depart the Hill Sphere of a body is actually slightly less than its theoretical escape velocity. This means any spacecraft reaching the Hill Radius with non-zero velocity will necessarily drift away from the Earth, at least at first. Furthermore, any perturbations from the Moon or other planets will cause unpredictable behaviour over time.

If the departure trajectory is in the plane of Earth's orbit, it may end up approaching one of the Earth-Sun Lagrange Points or eventually be recaptured by or collide with the Earth.

Perpendicular or normal trajectories are an interesting case. We will be in a slightly inclined orbit, but even if we ignore the Earth's gravity after passing the Hill Radius, we will still collide with Earth again after half an orbit when the two orbits cross.

All of these possibilities still leave the spacecraft in a very similar orbit to the Earth, regardless of the direction.

To achieve an orbit that is significantly different to Earth's, we will always need some velocity beyond escape velocity. This is know as hyperbolic excess velocity and is related to the Characteristic Energy.

Answer 3

This is a quick supplemental answer just to give additional confirmation to the other answers that for exactly v escape, the orbits remain similar to that of the Earth. Don't accept this answer!

I used Wikipedia's equation for escape velocity:

$$v_{esc}= \sqrt{\frac{2 GM}{r}}$$

and just integrated the forces of the Earth, Moon, Sun, and Jupiter on each other, and on six test particles. The six were fired straight "up" the +x, -x, +y, -y, +z, and -z directions from the corresponding six points on the Earth.

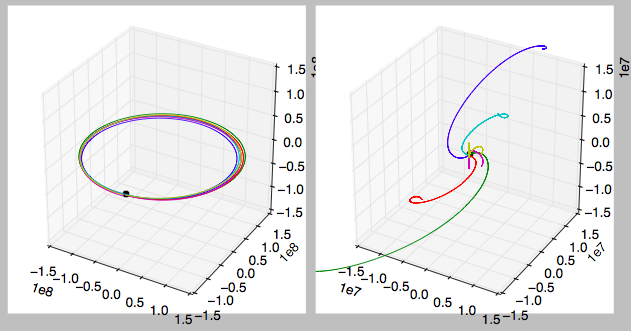
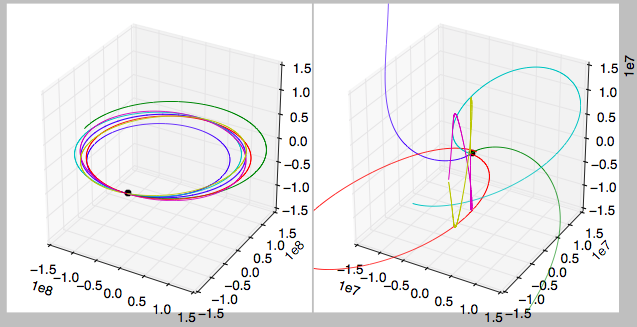
I ran it twice for 100% escape velocity (first plot), and 102% (second plot). The left side of each plot is in an inertial frame, the right side is in the synodic frame rotating with the Earth's orbital motion around the solar system barycenter, centered on the Earth.

I used the starting positions and velocities for the major bodies from June 29, 2018 00:00 UTC and JPL's Horizons

You can see this confirms what the other answers say. At exactly the escape velocity, the orbits are heliocentric and nearly the same as the Earth's. For 102% (and of course higher) they start deviating. You can see that the +/-z shots oscillate vertically.

Python script:

def deriv(X, t):
    x, v = X.reshape(2, -1)
    xx   = x.reshape(-1, 3)
    n    = xx.shape[0]
    accs = []
    for i in range(n):
        acc = np.zeros(3)
        for j in range(4):
            if j != i:
                xxij = xx[i] - xx[j]
                acc += -GM4[j] * xxij * ((xxij**2).sum())**-1.5
        accs.append(acc)
    accs = np.hstack(accs)

    return np.hstack((v, accs))

def rotatem(X, theta):
    cth, sth = [f(theta) for f in (np.cos, np.sin)]
    x, y, z = X
    xr = cth*x - sth*y
    yr = cth*y + sth*x
    return np.vstack((xr, yr, z))


import numpy as np
import matplotlib.pyplot as plt
from mpl_toolkits.mplot3d import Axes3D
from scipy.integrate import odeint as ODEint

GMe   = 3.9860E+14   # m/s
GMm   = 4.9049E+12
GMs   = 1.3271E+20
GMj   = 1.2669E+17
GM4   = GMe, GMm, GMs, GMj
R4    = 6378137., 1738100., 696392000., 71492000.   # m
names = 'Earth', 'Moon', 'Sun', 'Jupiter'

Re, Rm, Rs, Rj = R4

vescs = [np.sqrt(2.*GM/R) for (GM, R) in zip(GM4, R4)]
vesce = vescs[0]

for name, vesc in zip(names, vescs):
    print (name, vesc)
    
X0e  = 1000. * np.array([1.3539E+07, -1.5044E+08, -7.7480E+03,
                         2.9164E+01,  2.5290E+00,  8.3979E-04])

X0m  = 1000. * np.array([1.3481E+07, -1.5083E+08,  1.7757E+04,
                         3.0127E+01,  2.3628E+00, -6.1480E-02])

X0s  = 1000. * np.array([9.8486E+04,  1.0333E+06, -1.3866E+04,
                         -1.2308E-02,  6.41628E-03,  3.0230E-04])

X0j  = 1000. * np.array([-4.9851E+08, -6.3418E+08,  1.3781E+07,
                         1.0118E+01, -7.4520E+00, -1.9535E-01])

X0x  = np.hstack([x[:3] for x in (X0e, X0m, X0s, X0j)])
X0v  = np.hstack([x[3:] for x in (X0e, X0m, X0s, X0j)])

d = np.array(((-1, 0, 0), (1, 0, 0),
              (0, -1, 0), (0, 1, 0),
              (0, 0, -1), (0, 0, 1)), dtype=float)

xobs = (d*Re + X0e[:3]).flatten()

factor = 1.02
vobs = (d*vesce*factor + X0e[3:]).flatten()

X0x  = np.hstack((X0x, xobs))
X0v  = np.hstack((X0v, vobs))

X0   = np.hstack((X0x, X0v))

rs   = np.sqrt((X0x.reshape(-1, 3)**2).sum(axis=0))

for name, vesc, r in zip(names, vescs, rs):
    print (name, vesc, r)

times = np.arange(0, 365*24*3600, 10000)

answer, info = ODEint(deriv, X0, times, full_output=True)

n          = answer.shape[0]
xall, vall = answer.T.reshape(2, -1, 3, n)
xe,   ve   = [thing[0] for thing in (xall, vall)]
xps,  vpe  = [thing[4:] for thing in (xall, vall)]

theta = np.arctan2(xe[1], xe[0])

xer   = rotatem(xe,  -theta)
xpsr  = np.stack([rotatem(thing, -theta) for thing in xps])

if True:
    fig         = plt.figure()

    ax1 = fig.add_subplot(1, 2, 1, projection='3d')
    w   = 1.5E+08 

    x, y, z = 1E-03 * xe
    ax1.plot(x, y, z, '-b', linewidth=1)
    ax1.plot(x[:1], y[:1], z[:1], 'ok')

    for x, y, z in 1E-03 * xps:
        ax1.plot(x, y, z, linewidth=0.5)
    ax1.set_xlim(-w, w)
    ax1.set_ylim(-w, w)
    ax1.set_zlim(-w, w)

    ax2 = fig.add_subplot(1, 2, 2, projection='3d')
    w   = 1.5E+07 

    x, y, z = 1E-03 * (xer-xer)
    ax2.plot(x, y, z, '-b', linewidth=1)
    ax2.plot(x[:1], y[:1], z[:1], 'ok')

    for x, y, z in 1E-03 * (xpsr-xer):
        ax2.plot(x, y, z, linewidth=0.5)
    ax2.set_xlim(-w, w)
    ax2.set_ylim(-w, w)
    ax2.set_zlim(-w, w)

    plt.show()