30
$\begingroup$

Since NASA is once again targetting low-cost missions to the Moon, I was thinking about low-cost methods of getting stuff from the Moon back to Earth.

Although it seems reasonable to assume that anything sent to the Moon that we don't need back will simply be left there, I was wondering about the cheapest method of getting stuff back.

Let's say a cargo container filled with Lunar regolith, or t-shirts with a catchy logo ("NASA went to the Moon and all they sent back was this crappy t-shirt")

Assuming travel time is no real concern, could a single-stage rocket ejecting the vehicle from the Moon make the journey back to the Earth with no other propulsive aids, or would it simply get lost in space?

Is there a way that I could get my promotional t-shirt using the least possible amount of propulsion to get away from the Moon?

Improve this question
$\endgroup$
11
  • $\begingroup$ Related. $\endgroup$
    – leftaroundabout
    Commented Sep 4, 2018 at 8:56
  • 6
    $\begingroup$ @Uwe I was using humour to make the question a little bit more interesting. At its core, this question is an exploration of extreme low-cost transit from the Moon back to Earth. The actual cargo might well be more valuable, if you want to think about it that way. $\endgroup$
    – user21233
    Commented Sep 4, 2018 at 13:52
  • 7
    $\begingroup$ Isn't this pretty much what the Apollo missions did? $\endgroup$
    – jamesqf
    Commented Sep 4, 2018 at 14:43
  • 1
    $\begingroup$ @WGroleau: You don't need fuel (other than a small amount for course corrections) for re-entry from the moon. You just need to hit the atmosphere at the right angle, and have a good heat shield. See Apollo missions. $\endgroup$
    – jamesqf
    Commented Sep 5, 2018 at 4:41
  • 2
    $\begingroup$ Upvoted because I want one of those t-shirts if you manage to pull this off. $\endgroup$
    – Diego Sánchez
    Commented Sep 5, 2018 at 14:35

5 Answers 5

Reset to default
45
$\begingroup$

http://nbviewer.jupyter.org/gist/leftaroundabout/3955d27877e19be39d0f61fdafce069e

Barely achieving escape velocity means you take a parabolic orbit. The thing with parabolic orbits is that they actually approach zero speed as you depart to infinite distance from the starting body.

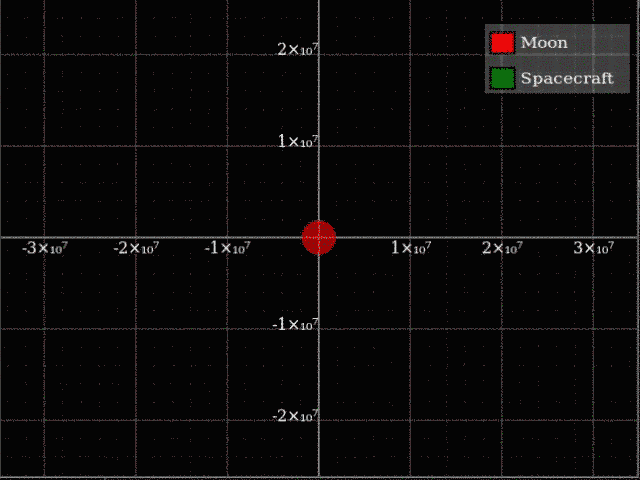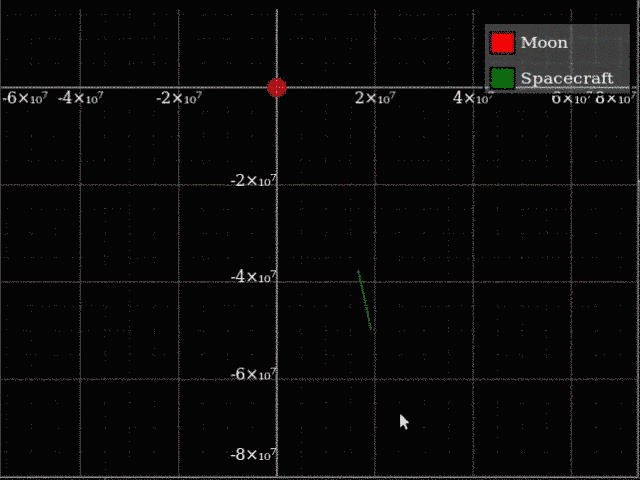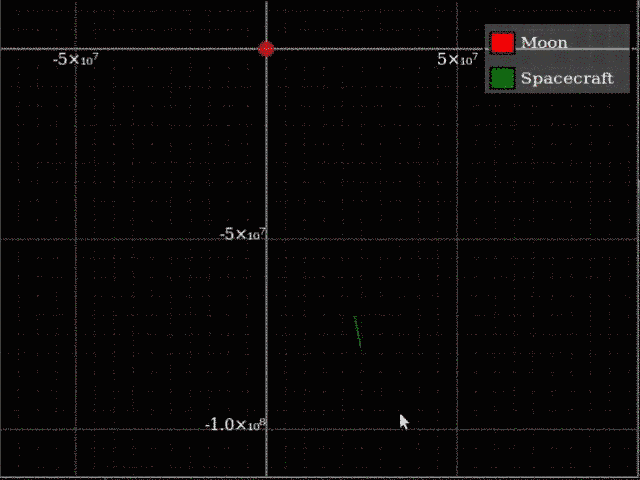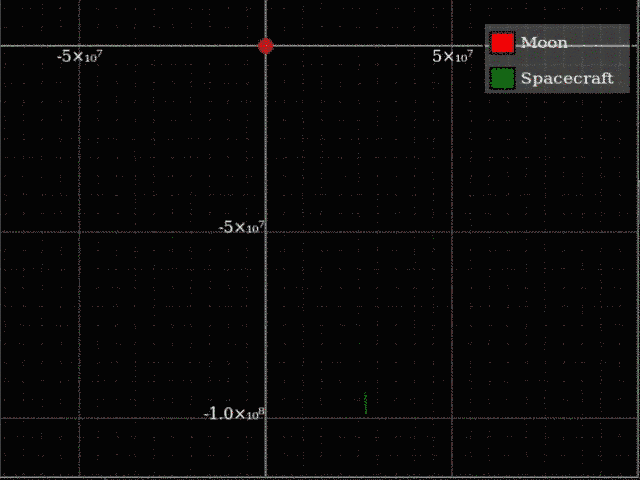
Animation of a parabolic escape orbit from Moon

That is, zero speed with respect to the starting body's frame of reference, i.e. in this case in the frame of reference of the moon. But, that's not zero speed in the frame of reference of the earth, or of the sun – seen from these, it's the same velocity as the moon itself. That's the reason the Parker Solar Probe required the huge rocket Delta IV Heavy: escaping earth was only part of the $\Delta v$, the interesting part is getting rid of the motion you inherit from Earth.

Actually though, parabolic orbits only exist in a true 2-body system. In reality, you don't keep reduce your speed to zero, because the Earth isn't that far away and immediately will influence the orbit. In particular if you start tangentially away from the forward-facing moon surface, heading away from Earth, then the retrograde-facing parabolic escape will give Earth time to “pull the spacecraft closer” while it has less than the Moon's speed. As a result, the orbit will actually have a substantially lower perigee than the Moon:

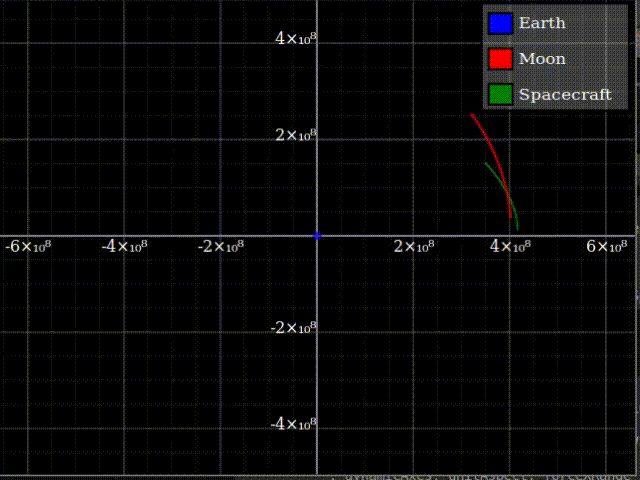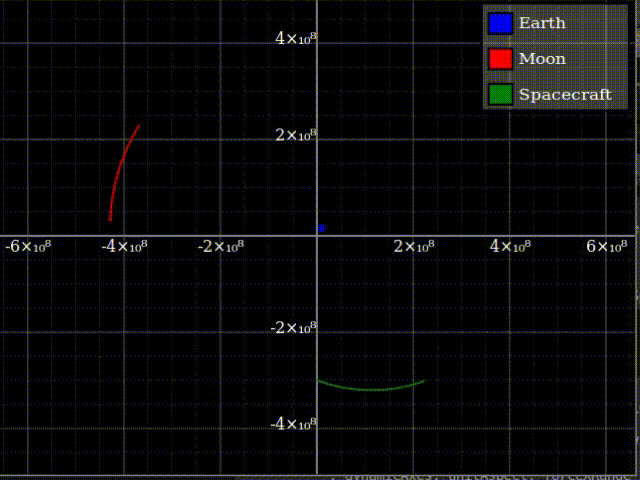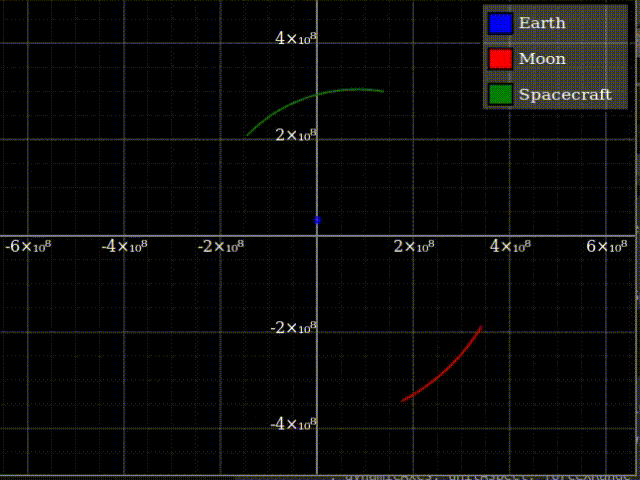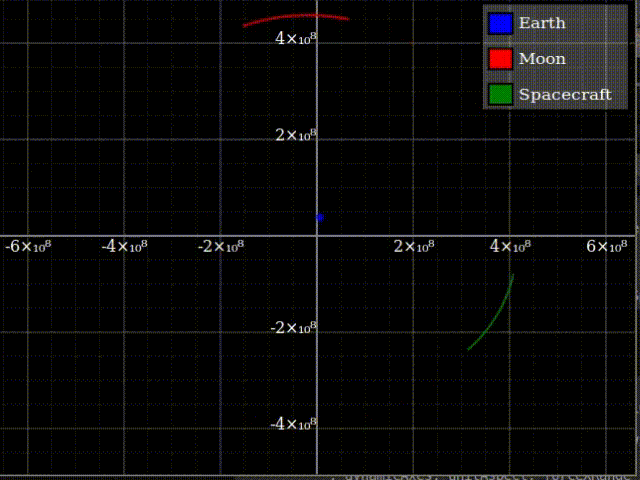
How a just-escape orbit from moon might actually look

You could now cleverly fine-tune this so after four orbits or so, you get another close approach to the moon that will then slingshot you right into Earth.

But since neither the Moon or Earth are very massive, it's actually more practical to just pack in some extra $\Delta v$, to start out with a hyperbolic trajectory from the moon. Example with $v_0 = 2572\mathrm{\tfrac{m}s}$ (escape velocity is $2375\mathrm{\tfrac{m}s}$):

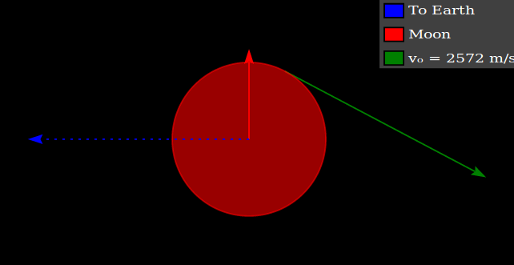
Launch vector of crash-headed Moon start

Crash orbit

View of the same trajectory from the Moon:

Hyperbolic escape orbit that crashes on Earth

Sorry for the bad quality GIFs, I can't seem to get them reliably optimised to be accepted by imgur in any other way.

Improve this answer
$\endgroup$
10
  • 3
    $\begingroup$ Nice. So it adds less than 200 m/s to lunar escape to get directly onto an Earth intercept. $\endgroup$
    – Steve Linton
    Commented Sep 4, 2018 at 13:43
  • 3
    $\begingroup$ Beautiful answers like this is why I like visiting the Space Ex stack so much. Thank you very much! $\endgroup$
    – user21233
    Commented Sep 4, 2018 at 15:30
  • 1
    $\begingroup$ @uhoh yeah, it fires $2275\mathrm{\tfrac{m}s}$ away from the Earth and $1200\mathrm{\tfrac{m}s}$ in retrograde orbit direction, starting from the spot on the far side of the Moon where this vector is tangential to the surface. (Not sure why I used this sign convention...) — I haven't exhaustively checked how much lower we could make the $|v_0|$, but I don't think it can be much less. Most of the similar combinations just give a highly elliptical geocentric orbit – low periapsis and Moon-like apoapsis. As long as we exceed the Moon's escape velocity, it doesn't go back to the Moon at any rate. $\endgroup$
    – leftaroundabout
    Commented Sep 4, 2018 at 16:58
  • 1
    $\begingroup$ @leftaroundabout Regarding the image quality, maybe using APNG instead of GIF would help? Although IE and Edge users might be left out, then. $\endgroup$
    – 8bittree
    Commented Sep 4, 2018 at 18:39
  • 2
    $\begingroup$ Those animations are outstanding! I'm really impressed by this answer overall. $\endgroup$
    – Lightness Races in Orbit
    Commented Sep 5, 2018 at 11:18
15
$\begingroup$

It would be lost in space.

If you barely reached the moon escape velocity, it means that your object will reach an orbit somewhat similar to that of the moon.

From there, the orbit will be unstable due to earth/moon (and other bodies) interactions. It might take the cargo back earth, back to the moon, or in deep space. Predicting accurately theses orbits is difficult, and unreliable on the long term.

Unfortunately, this doesn't seems like a practical solution.

You can read more about delta v budgets on wikipedia.

Improve this answer
$\endgroup$
8
  • 10
    $\begingroup$ It would be (most likely) lost in space (if it were not launched carefully). But if it were done carefully, wouldn't there be some trajectories that would indeed lead to a close approach to Earth for capture, or even a reentry? Or is there a mathematical argument based on $C_3$, manifolds, etc. that would preclude this? You might be right, but a good answer should include some supporting information or argument. That way future readers can learn something more than "Antzi doesn't think so." $\endgroup$
    – uhoh
    Commented Sep 4, 2018 at 7:19
  • 1
    $\begingroup$ @uhoh I agree this is a half answer $\endgroup$
    – Antzi
    Commented Sep 4, 2018 at 7:51
  • 1
    $\begingroup$ @uhoh: The quality issue isn't in the answer, it's that OP's question leaves many specifics out (such as the direction in which you leave the moon's SOI). You can't succinctly answer every possibility that the question has left open. The most efficient (pound for pound) would be to exit the moon's SOI in the moon's retrograde direction. However, the issue in OP's question is "barely exceeding". You still need a fair amount of energy to lower your orbit for capture. The closer you get to barely escaping the moon's SOI, the less likely you are to actually get captured by Earth. $\endgroup$
    – Flater
    Commented Sep 4, 2018 at 10:32
  • 1
    $\begingroup$ I think with very precisely executed departure, it could grab some braking Moon assists later - and eventually get a reentry trajectory that way. But obtaining these without budget for corrective burns would be very difficult. $\endgroup$
    – SF.
    Commented Sep 4, 2018 at 12:31
  • 3
    $\begingroup$ @Flater: For the parts that were not specified assume freedom - pick the most convenient you want. $\endgroup$
    – SF.
    Commented Sep 4, 2018 at 12:34
7
$\begingroup$

Barely achieving lunar escape velocity means when you stop thrusting you're now on an elliptical orbit that overlaps the moons orbit but certainly doesn't dive deep enough in the earth/moon system to actually be captured by earth's atmosphere.

You'll orbit that way until the moon comes back around and you do one of three things.

  • Crash into the moon
  • Get your orbit slingshotted into an earth-intercept where you burn up in the atmosphere
  • or slingshot into an earth/moon escape and solar orbit.

The odds of that slingshot effect getting you to earth safely are pretty minimal.

Source: Repeatedly had it happen to me while time-lapsing in Kerbal Space Program

Improve this answer
$\endgroup$
5
  • 3
    $\begingroup$ If the second option (Earth-intercept) is possible at all, then it should be possible to achieve it by careful timing and direction of the initial launch from the Moon, followed by small trajectory corrections. The payload would launch from the Moon and then orbit for a fairly long time (probably) before starting a series of perigee-lowering slingshot maneouvers around the Moon (a little like the Parker solar probe and Venus) eventually resulting in Earth intercept. It would take a while but the total delta-V budget should be small. $\endgroup$
    – Steve Linton
    Commented Sep 4, 2018 at 9:18
  • 1
    $\begingroup$ It would be extremely chaotic due to the interactions of the earth/moon system, but yes, technically if you had the benefit of sufficient computing power you could do it. your spacecraft will ultimately need to produce a dV of around 3km/s to reach a trajectory that intercepts the earth, some of that can come from the slingshot. You'll also have to survive an 11km/s reentry velocity, but you can perform multiple aerobraking passes because you don't care about time. so that's less of an issue, just graze the atmosphere repeatedly to lower your apogee until you reenter. $\endgroup$
    – Ruadhan2300
    Commented Sep 4, 2018 at 9:38
  • $\begingroup$ @Ruadhan2300: How much CPU power would be needed to get within e.g. a factor of 2 of optimal delta-V? I would think that in most cases where it would be hard to determine which of two courses of action would be better, both courses of action would be almost equally good. $\endgroup$
    – supercat
    Commented Sep 4, 2018 at 17:07
  • $\begingroup$ I'd say precision on your flight-plan is the main requirement, you need to work out an N-Body approximation of the earth and moon system and track your trajectory routinely to make sure it's in line with expectations. Asking how much CPU is probably not useful, you have months between swing-bys to perform the calculations, you could probably do it on paper.. Optimal dV after reaching escape-velocity should be negligible if you get the initial trajectory just right but the flight-time is certainly going to be measured in years. I'll give it a try in KSP tonight after work :) $\endgroup$
    – Ruadhan2300
    Commented Sep 5, 2018 at 8:31
  • 2
    $\begingroup$ +1 for using KSP as a source. NASA only do that when they get really stuck. :) $\endgroup$
    – Wossname
    Commented Sep 6, 2018 at 8:29
6
$\begingroup$

If you take the eliptical orbit from the Moon surface, then no, at the escape point your speed will hardly differ from that of the Moon and your sample will orbit there indefinitely.

Buuut.... if you escape the Moon from the highest possible retrograde Moon orbit...

Moon hill sphere radius: 58120 km

Orbital speed around the Moon there: 0.29km/s src

Your orbital speed will be orbital speed of the Moon, minus your orbital speed.

Escaping, with infinitesimal burn, in retrograde direction, at the far point, you'll land in Earth orbit, at apogee of 442500 km src

1.02 km/s of Moon orbital velocity - 0.29km/s = 0.7km/s.

Using Vis-Viva equation, we get 304,000 km of semi-major axis.

Now, apogee+perigee = 2x semi-major axis, so 2 * 304,000 km - 442500 km = 165500 km...

...and bummer. At 165,000km we won't even get any trace aerobraking.

Improve this answer
$\endgroup$
2
  • $\begingroup$ Nice answer but rather than “Barely above escape velocity” I think op meant overall smallest delta v from the surface $\endgroup$
    – Antzi
    Commented Sep 4, 2018 at 16:44
  • 3
    $\begingroup$ @Antzi: Op didn't specify 'from the surface' and even if s/he meant that the answer would be boring. $\endgroup$
    – SF.
    Commented Sep 4, 2018 at 22:43
4
$\begingroup$

It should be possible, given enough time and very careful navigation. You might need to do a little better than "barely" escape, but you would not need to propulsively lower your Earth periapsis significantly below the Moon.

Over a period of years, possibly many years, you would need to arrange for swingbys of the Moon to crank up the eccentricity of your Earth orbit until you enter the Earth's atmosphere.

How long it would take would depend on how often you are able to reencounter the Moon. That is where you would want to not "barely" escape, since that would increase the time between reencounters.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.