Why is L2 Langrangian point stable?

Question

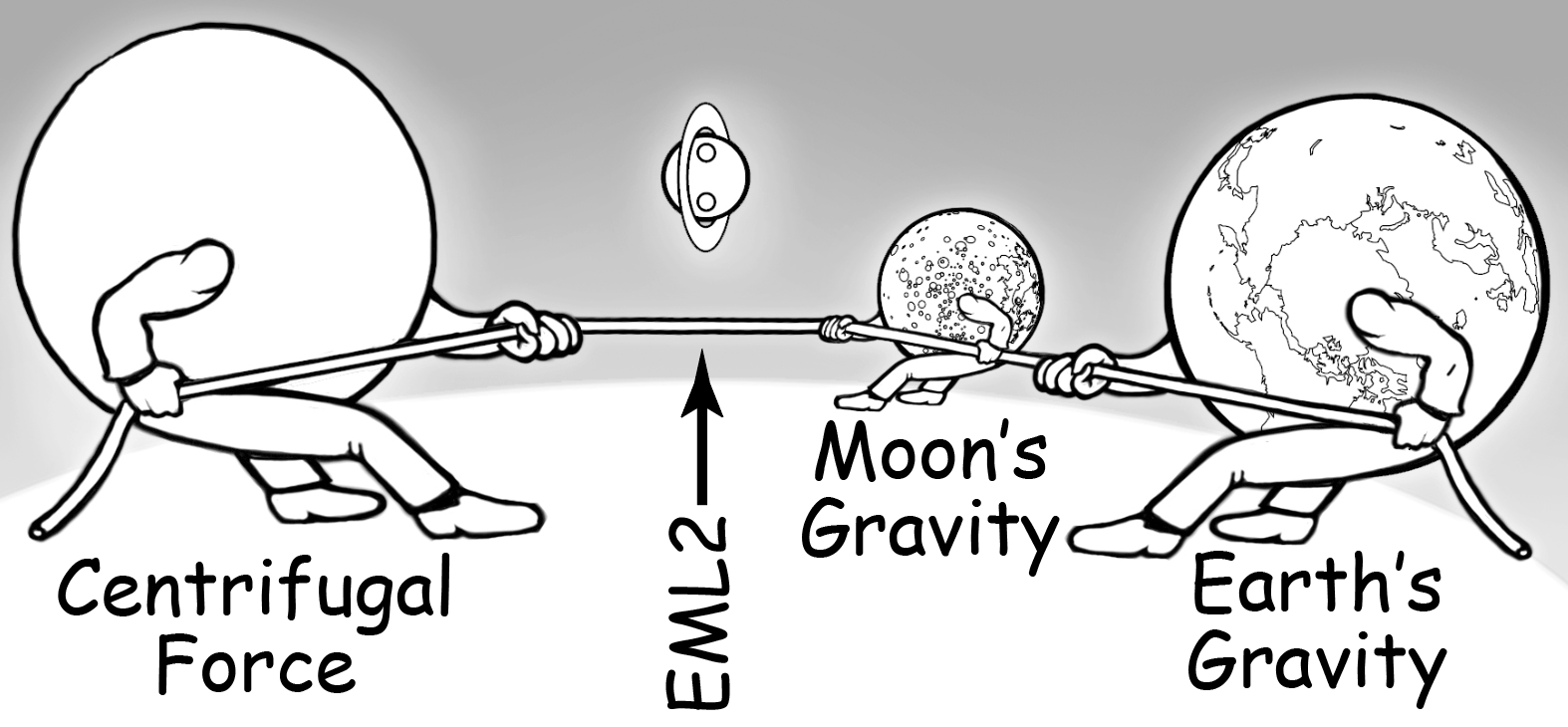
I was reading about Langrangian points between Sun and Earth. I understood that $L_1$ Langrangian point was stable as the gravitational pull by Sun on the satellite towards itself was equal to the gravitational pull by Earth towards itself. Then I came across these two paragraphs (you can check it here)

L2 is located on the same line as the mass but on the far side. So, you’d get Sun, Earth, L2 point. At this point, you’re probably wondering why the combined gravity of the two massive objects doesn’t just pull that poor satellite down to Earth.

It’s important to think about orbital trajectories. The satellite at that L2 point will be in a higher orbit and would be expected to fall behind the Earth, as it’s moving more slowly around the Sun. But the gravitational pull of the Earth pulls it forward, helping to keep it in this stable position.

Now, I understand that planets in outer orbits revolve around the Sun slower than inner orbit planets, but how is slow speed reversing the nature of gravitational force of Sun on the satellite, I mean, like $L_1$ position experiences inward pull by the Sun, why then, $L_2$ point is experiencing outward push from the Sun? There should have been net inward pull by Earth and Sun together in the same direction.

Also, in the two paragraphs which I first mentioned, they talked about higher orbits. Now, what exactly is higher orbits? Higher in height relative to other orbits?, Or more farther in horizontal distance from the successive orbits around the Sun (if we consider all orbits lying on a horizontal plane)? . Can someone please explain these doubts?

Answer 1

Gravity does not cancel out at any of the Lagrange points, not even L1. As you point out, at L2 both the central body and orbiting body are pulling the same direction.

But there is a third character in this tug of war, centrifugal acceleration. It's not truly an acceleration but inertia in a rotating frame. But when you're in the rotating frame it sure feels like you're being pulled outward.

Here is a diagram of EML2 (Earth Moon L2) from my page 51 of my orbital mechanics coloring book:

I talk about this more at Lamentable Lagrange Articles

Answer 2

At the L2 point, the satellite does feel the inward pull of the Earth and the Sun together in the same direction, just as you think it should. That point is chosen to be at the distance so that the combined gravity of the Sun and Earth is just strong enough to keep the satellite in an orbit that takes just one year. If the Earth were not there, then the speed required for a circular orbit would be less, and it would take more than a year. At that distance and that speed, Earth just keeps up, so it always has the right gravitational force on it to keep it in that same orbit.

Imagine putting a satellite in orbit about the Sun, but somewhere between Earth and the L2 point. At that distance, the combined gravity of the Earth and Sun would have the satellite orbiting in less than a year, so it will start to get ahead of the Earth. As it gets farther from the Earth, the effect of Earth's gravity will be less and less, and so your satellite won't stay in a circular orbit. It will still be approximately circular, but it will be perturbed by the Earth every time the two approach the points in their orbits where they are nearest each other.

(For simplicity, I described this as if the Earth's orbit were circular. I don't know the details of actually taking into account the main orbit being non-circular.)