As a continuation of my previous post regarding the use of the software GMAT (General Mission Analysis Tool) (website, YouTube) for propagating spacecraft, I have an additional question.
This time I propagated 2 spacecraft in a $J_2$ gravity field (gravity degree 2 and gravity order 0, no higher gravitational harmonics, no drag, no sun radiation pressure, no third bodies). Both spacecraft have the same initial mean orbital elements: $$[\bar{a}_0,\bar{e}_0,\bar{i}_0,\bar{\Omega}_0,\bar{\omega}_0,\bar{M}_0] = [7000\text{ km}, 0, 45 \text{ deg}, 0, 0, 0]$$ The only difference between the spacecraft is their epoch. The first spacecraft epoch is $$1/1/2005 \quad \quad 00:00:00$$ The second spacecraft epoch is $$1/1/2015 \quad \quad 00:00:00$$
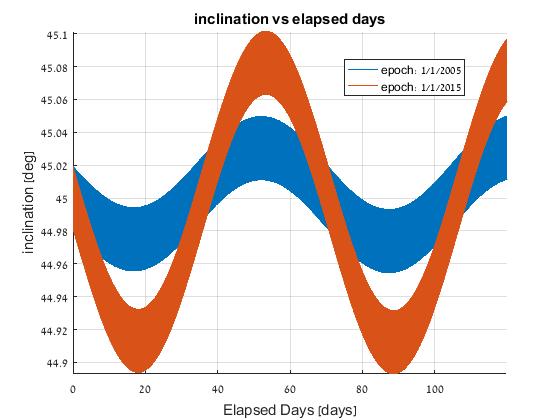
Finally, I plotted their osculating inclinations in the J2000 frame (The regular Earth Centered Inertial (ECI) frame):
The $J_2$ induced long-period inclination oscillation (period of about 70 days) does not surprise me. What is surprising is that the year governs the oscillation amplitude.
Two points:
- To the best of my knowledge, the utilized gravity field is axisymmetric and time-constant, so no time-varying perturbation should occur.
- In order to verify this result, I also used STK with the same scenarios and got the same results.
I think that this phenomenon (year governing the oscillation amplitude) stems perhaps from precession and nutation effects. What do you think?
Update:
Here is an output from STK where I defined some initial conditions with the aforementioned force model:
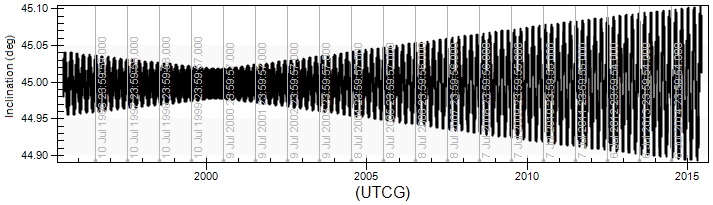
The plots depict the osculating inclination as a function of time. We can clearly see that on long time periods, the oscillation amplitude varies. Can anyone explain this dependency?