7
$\begingroup$

I'm only just beginning to scratch the surface of the possibilities in interstellar travel. I know that pulsars can be independently identified by means of measuring their pulses, and that once enough have been identified they can be used to identify one's position in space.

My question is: How long (on average) would a pulsar need to be observed in order to identify it from its pulses alone? More to the point, how long would a craft need to watch the pulsars it finds to identify them and get a "fix" on its location based on that? (That is, is the distribution uniform, or is it skewed one way or the other and, if so, how does that affect the average time required to identify enough pulsars?)

For the purposes of this question, we're assuming highly accurate observational and measuring tools and equipment, rather than a bearded old man with a telescope in his backyard making all his calculations by hand.

I suppose another way to ask the same thing would be: What's the average cycle duration of pulsars, and how much skew is there to the distribution?

Improve this question
$\endgroup$
11
  • 4
    $\begingroup$ This is incredibly dependent on how much and how good your tech is. If you have a million cameras on the outside of your ship that can record light levels with 64-bit precision you'll find yourself in seconds. If you have a geezer with a telescope and a spinning disk with a hole to measure pulse rates it's going to take years. $\endgroup$
    – Loren Pechtel
    Commented Apr 25, 2014 at 16:40
  • $\begingroup$ @LorenPechtel That's a very good point. Let's assume for the purposes of this question the absolute best observational tools and techniques science and technology have provided us. (The genesis of the question is my research for a hard-sci-fi novel involving interstellar travel, and I want to as much as possible avoid meaningless technobabble while still providing realistic explanations for how it all works.) Of course, an answer encompassing both ends of the spectrum would certainly get my up-vote, and The Green Checkmark! $\endgroup$
    – Kromey
    Commented Apr 25, 2014 at 16:45
  • $\begingroup$ I still don't think you can get a good answer because you can always add more cameras until you run out of hull. It's a problem that's subject to parallelism--how many sensors do you want to carry around. $\endgroup$
    – Loren Pechtel
    Commented Apr 25, 2014 at 21:25
  • 1
    $\begingroup$ And still another unknown: How unknown is your location? If your stardrive can't hop you more than 10ly then once you find a pulsar you have substantially narrowed your search for additional pulsars--and each additional pulsar narrows the search more. On the other hand, if your drive is good for 10,000ly the first pulsar tells you nothing about where others might be. It will also be much harder to identify pulsars if you can go that far--pulsars are NOT consistent through time and thus you'll get more cases of a signal that you can't tell which pulsar it is until you have more data. $\endgroup$
    – Loren Pechtel
    Commented Apr 25, 2014 at 21:30
  • 2
    $\begingroup$ Since this question received one close vote as off-topic, I would like to argue that astronavigation is on scope here as it is directly relevant to spacecraft operation. We've previously discussed star trackers, Polaris equivalent stars on Mars, and so on here on Space Exploration. $\endgroup$
    – TildalWave
    Commented Apr 29, 2014 at 16:43

1 Answer 1

Reset to default
2
$\begingroup$

Here's a correct answer: A craft couldn't.

We haven't mapped the whole of space yet so...

a craft with no other means of identifying its position

... can't know if it's near known or unknown pulsars. If you really have no idea of where you are or what your attitude is then looking around you isn't actually that useful. For example, you wake up in a forest, and from the flora and fauna you surmise that you must be in the Amazon rain forest. But you could easily be on another planet, in another galaxy, far far away.

Now if you have knowledge of your approximate position, say somewhere in the milky way, then you can start using pulsars and other things you see in space to calculate your position. You would need three known pulsars to triangulate your position (with 2 pulsars you can only know you're in 1 of 2 possible locations). If you know enough about the pulsars you could do some interesting red shift calculations to find out how far away from them you are. When it comes to the actual identification process, the duration of observation required is dependant on how many pulsars it might be, based firstly on your expected position and then on initial observations.

For example if you see a pulsar and you think you're near 1 of 10 pulsars then you can assume the pulsar you're seeing is one of the 10. Then you observe the pulsing of the pulsar and see at what rate it pulses. The longer you observer the pulsar the more accurate you calculation of it's spin can be, and hence the easier it is to rule out the other 9 pulsars.

EDIT:

The reason for a simple answer not being given to this question (by me at least), is that it is highly dependant on which pulsars you are looking at. For example take the following collection of pulsars and pulse frequencies:

A  0.98
B  0.12
C  0.51
D  0.78
E  0.15
F  12.01

It's clear that if you are trying to identify pulsar F it is going to be an easy task. If you see more than one pulse per second you can be confident that the other pulsars are ruled out. Now consider the following collection:

A  13.52
B  12.08
C  10.54
D  15.23
E  11.98
F  12.01

To identify pulsar F in this collection you Need to be able to observe the pulsar for around 13 seconds to rule out pulsar B as a potential candidate. This assumes that you can only count the number of pulses in a given number of seconds. This is unlikely, but there will be a limit to how accurately you can time pulses, dependent on the design of your hardware.

To surmise, the duration required to identify pulsar F in collection 2 is 13 times that of collection 1; this is why it's dependent on the number and characteristics of pulsars in range.

EDIT 2:

The following is based on values for 1,861 pulsars.

median: 1.88 Hz
highest: 716.35556 Hz  (PSR J1748-2446ad)
lowest: 0.084897259 Hz  (PSR J1841-0456)

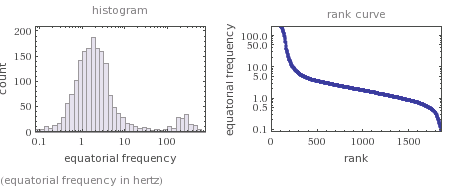
Distribution plots

This looks to me like a large number in the 1 - 4Hz range say around 700. So if the values are evenly distributed in this range there is approximately 0.004hz between each pulsar's equatorial frequency. The longest frequency involved in this large subset is 1Hz, which suggests that if you can measure to the nearest 0.004Hz then you can identify the majority of pulsars in 1 second or less. If your measuring accuracy is lower this will increase the time require.

Reference: The data is a composite from 52 published works. A list can be found at the bottom of the page HERE, under external sources.

Improve this answer
$\endgroup$
19
  • $\begingroup$ Would you mind providing a few references? $\endgroup$
    – Deer Hunter
    Commented Apr 28, 2014 at 11:39
  • 2
    $\begingroup$ He's assuming some sort of stardrive, I don't think it's reasonable to declare it impossible based on a current lack of mapping. $\endgroup$
    – Loren Pechtel
    Commented Apr 28, 2014 at 18:41
  • $\begingroup$ @DeerHunter I don't have any references. This is all just logic. $\endgroup$
    – ThePlanMan
    Commented Apr 28, 2014 at 19:23
  • $\begingroup$ @LorenPechtel I'm assuming no such thing. I'm basing my answer on the specifics of the question. It would be a similar scenario if you were to awake from some sort of cryogenic storage. We as a species are not sure if the universe is infinite (current thinking is now leaning towards it being infinite), so no matter how well you map space, an infinite universe will always have an infinite percentage unmapped. :) $\endgroup$
    – ThePlanMan
    Commented Apr 28, 2014 at 19:26
  • 2
    $\begingroup$ @FraserOfSmeg Correction: When knowing the angle between two points in three-dimensional space but not your own orientation, you would know that you are on the edge of a circle, not on one of two points. $\endgroup$
    – Philipp
    Commented Apr 29, 2014 at 8:08

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.