What is the delta V required to access a polar lunar orbit?

Question

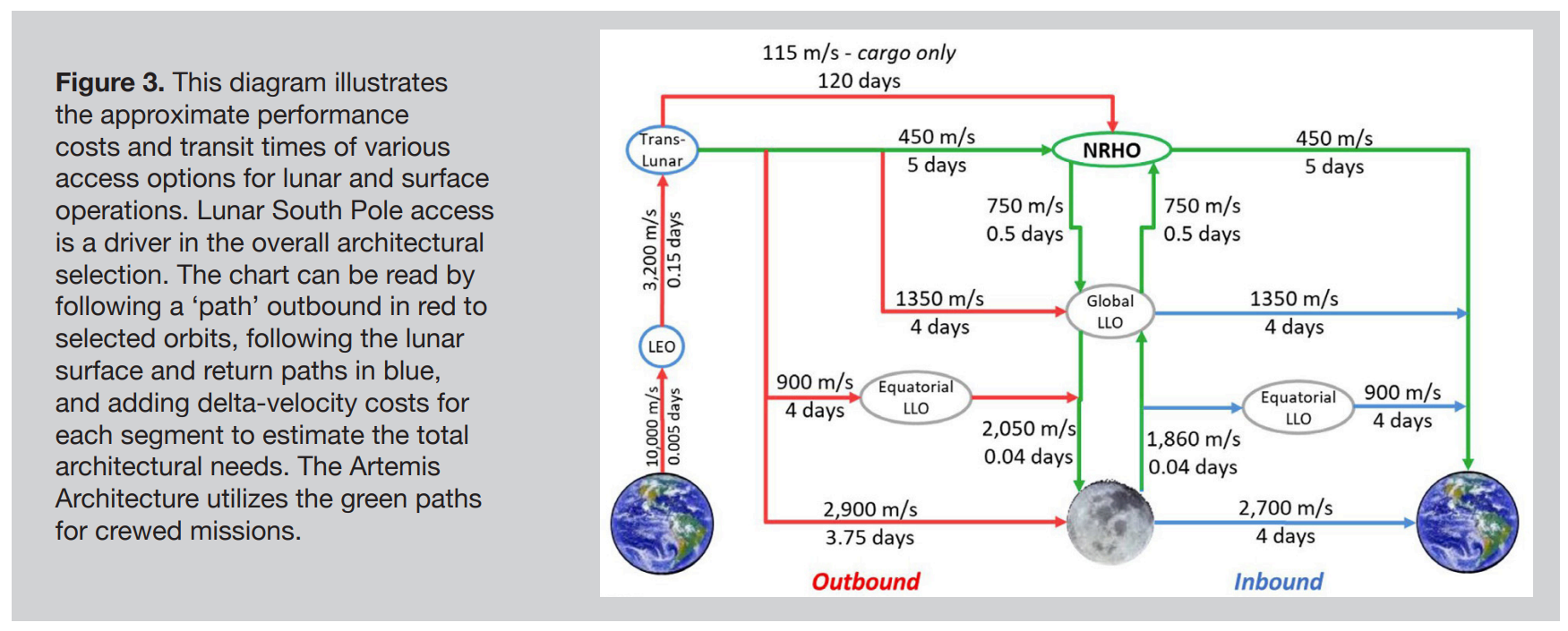
The general reference figure (I use) for entering a low lunar orbit (polar included) is ~900 m/s, which is roughly what was set out in previous questions. However NASA released this white paper which included this cislunar delta v chart showing the cost to enter a polar low lunar orbit being 1350 m/s (which is actually more expensive than the indirect NRHO path shown).

So this raises the question of what the actual range of Δv's for reaching a 100km circular polar LLO is? It doesn't seem that consistent. What drives this range? I hear the term 3-body physics and rotating frames and they scare me.

Also a somewhat separate follow up question, is the exit delta v to return to Earth from the polar LLO relatively constant or does it change by a fair bit based on phase?

Answer 1

Basically, you are right in saying that the Δv is not very consistent across timeframes. Why? Well, Δv is basically a proxy for the energy requirement for a maneuver, or at certain points, and this is crucial, the work done against gravity to get to a certain point.

Now why is this important? For near-Earth objects such as satellites, the primary force is gravity of the Earth. However, at some distance from Earth, the forces from the Moon (and to a lesser level the Sun) reach a level comparable to that of Earth. This means that a relatively small burn here will bring about a much larger change in direction than say near the Earth's surface.

Now onto the mission planning aspect. There are competing needs when planning a mission. Obviously, for a rover/orbiter, there is no rush to get to the moon ASAP(think lunar missions with 3+ month rendezvous time). Here, it makes sense to minimise cost and delta-v budget in exchange for a longer time. As such, by considering the relative positions of planets and the Sun a much longer(in terms of time) but cheaper(in terms of delta-v budget) can be achieved.

On the other hand, a manned mission, because of different needs(e.g. inability to bring very large amounts of food), needs to get to the moon and back much faster, as such mission time is prioritised with direct Hohmann transfers, increasing delta-v.

You might be interested in looking at Weak-Stability Boundary and the Interplanetary Transport Network for a more indepth discussion of how such low delta-v missions are planned.