Let's say you have a spaceship on its way to Mars; halfway there you decide you need to come back to Earth instead.
Is there any reason a spacecraft would be unable to do this? Let's assume it began in Earth orbit with enough fuel/engines to leave orbit and head for Mars, and that it was already planning to come back after orbiting Mars.
Let's say you start rolling down from the top of a half-pipe skateboard ramp, and you plan to get back to your starting point. If you stop in the middle of the pipe, it is much harder to climb back up to your starting point then if you ride up the other side of the ramp and let gravity accelerate you back.
Similarly, even if you planned to get into an orbit in Mars, most orbits are chosen so you only expend a small amount of energy decelerating to get into orbit and only expend a small amount of energy to leave the orbit and slingshot back. Compare this to stopping your spacecraft mid-space and accelerating back. After all, you conserve the momentum your spaceship has while orbiting around the planet.
All the other answers are great, but I think one explanation is still missing: how an interplanetary orbital transfer actually works in practice.
The thing is, space is rather big, and things keep moving. At the same time, you're being tugged on constantly by all the other bodies in a planetary system (we can ignore other stars for interplanetary transfers). So travelling between planets is not as easy as pointing your ship in the direction of planet B and pressing the speed pedal.
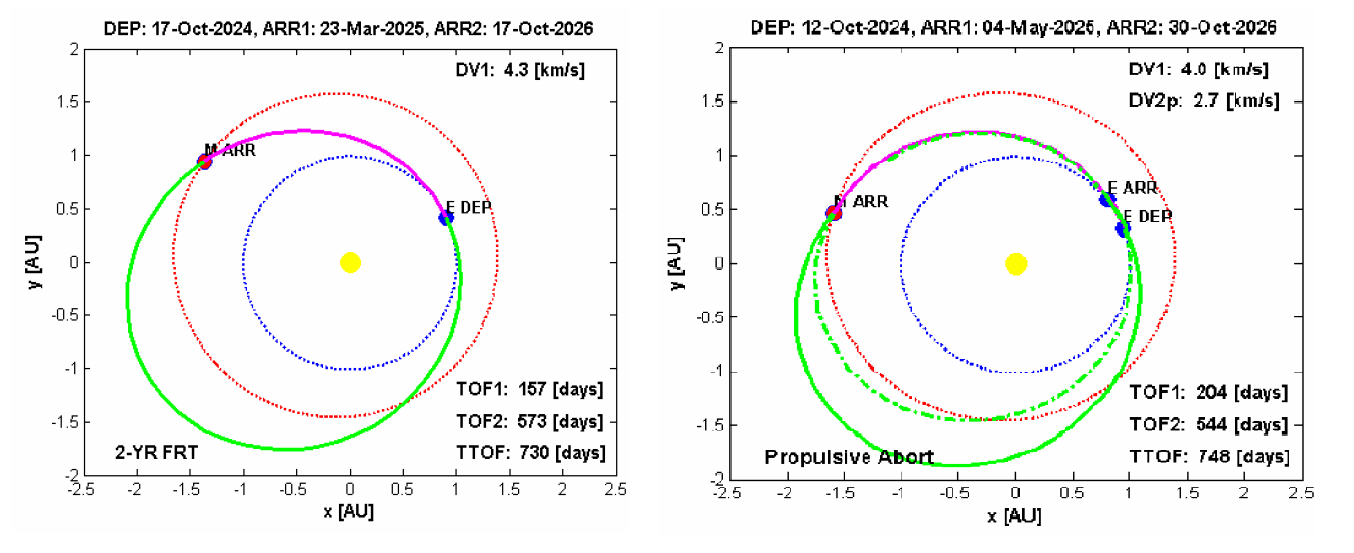
So, things keep moving. The historic Mars missions have taken anywhere from about 100 days to more than 300 days - the latter actually meaning that by the time you arrive, Mars is on the opposite side of the Solar system compared to when you started. You need to aim for where Mars will be, rather then where it is. Now, this isn't too big of a problem for the trip itself, but think about what happens when you abort at some point during the trip - the Earth has moved as well. It's not as easy as pointing your spaceship in the opposite direction and accelerating again - the Earth is no longer where it was when you started.
Why does it take so long to get to Mars? Well, space is big. Those probes were usually travelling at around 20 000 km/h - quite fast in terrestrial terms. But the closest distance between Earth and Mars is still over fifty million kilometers. The only way to get there faster is to get your speed up. Here lies the main problem - our spaceship engines are actually extremely inefficient. Jet engines are much less efficient than car engines, but they're still peanuts to rocket engines.
There's two main reasons - propellant and fuel. When you drive your car, the wheels are pushing of from the Earth - basically, you're either "stealing" or "giving" momentum to Earth, which means your car only needs to produce enough energy to supply your kinetic energy, give or take. With 100% efficiency in a vacuum, a 1 ton car would only need about 300 kJ of energy to accelerate to 100 km/h - that's about 10 grams of gasoline worth of energy. The first annoying thing is that kinetic energy grows quadratically with respect to speed - so accelerating to 200 km/h takes around 1400 kJ of energy, rather than 600 kJ. So getting even to that 20 000 km/h would take around 1400 MJ of energy (1.4E10 J) - the equivalent of about 280 kg of gasoline, which is more than a quarter of the mass of the car itself.
A quarter of the mass is quite a lot - a spaceship can't just stop every few kilometers to refill its fuel tank. It needs to carry all of its fuel from the very beginning - so our sample car wouldn't actually be 1 ton, it would be 1 ton + 280 kg of gasoline. And those 280 kg of gasoline would need even more gasoline to accelerate itself - this is known as the tyranny of the rocket equation - the more fuel you bring, the more fuel you need to bring the fuel, etc. Now, if we actually had a rocket that would be able to accelerate 1 ton to 20 000 km/h with just 280 kg of fuel, we'd be peachy. But we don't have anything close to that. Why? The two reasons mentioned above - fuel and propellant.
You might be saying, wait, we already accounted for fuel - that's the 280 kg of gasoline (plus the gasoline for the gasoline, ...), right? Nope. Gasoline doesn't just spontaneously break down, releasing energy - it's being burned, which is just a simple way of saying it chemically interacts with oxygen. Your car can easily use the ambient oxygen, getting its mass for free, but rockets tend to travel in a vacuum - they need to bring their oxygen along for the ride as well. That's extra mass, of course. How much mass? Well, using a very simplistic calculation (considering pure octane burning perfectly), you need about 3.5kg of oxygen to burn 1kg of gasoline. I think you can see the problem now - while we can barely ignore the 280kg compared to our 1 ton car, the problem becomes glaringly obvious when you actually need more fuel than your vehicle weighs (280kg of gasoline + 980kg of oxygen)!
But wait, this isn't over yet. This explains the fuel part of the issue, but there's still the propellant. Cars don't need propellant, since they're pushing off the ground. Even jets don't need to carry their own propellant, since they're again using ambient air as both fuel and propellant. But again, our rocket engine doesn't have the same luxury. It needs to carry its propellant along the trip. This is the place where rocket science gets really complicated (and we didn't even glance on orbits or interplanetary transit yet!).
Conservation of momentum basically says that to accelerate in one direction, you need to accelerate something else with the same mass in the opposite direction with the same acceleration. You can also trade mass for velocity - the faster you throw away your propellant, the less mass does it need to have to give you the same acceleration. This is the exhaust velocity of a rocket - the speed of the propellant relative to the rocket. To give you a bit of a scale, a typical liquid oxygen+liquid hydrogen rocket has an exhaust velocity around 18 000 km/h. This basically means that to accelerate a 1 ton spaceship to 18 000 km/h, you need 1 tons of propellant (ignoring the mass of the fuel and propellant itself and the mass of the engine). If we stop ignoring the mass of the fuel and propellant, we need to turn to the rocket equation - again, you need more fuel to accelerate the fuel.
The rocket equation has the following unknowns:
Putting the known values in our equation gives us an initial mass of about 3.2 tons - so you need about 2.2 tons of fuel to get your 1 ton spaceship to 20 000 km/h. That's already pretty bad - don't forget that we also need to get the spaceship into orbit in the first place, where the payload/fuel ratio is even worse. But wait, it gets even worse. To stop the ship, you again need to accelerate the same amount. Another 5 500 m/s brings us to a fuel mass of 9.3 tons - and that's just stopping at the target. To enable a mid-transit return trip, you need 5 500 m/s for the initial acceleration, 5 500 m/s to stop, 5 500 m/s to start back again and 5 500 m/s to stop at the end. This gives you a monstrous 22 000 m/s delta-V requirement, requiring 106 tons of fuel for your tiny 1 ton spaceship. And that ignores the mass required to actually store all that fuel, as well as all the fuel lines etc. needed to get it to the engine!
It should be obvious that with our current rocket engine technology, what you're proposing is pretty much outright impossible. There's two ways around this - one is staging (throwing away "spent" parts of your spaceship), one is keeping your delta-V requirements as low as possible.
Staging allows you to build rockets with higher fuel/ship mass ratios. For a single stage rocket, a ratio of about 15 is already pretty difficult, and anything above 20 is very unrealistic given our technology. This is mostly due to the fact that you need all kinds of supports etc. to make sure your rocket doesn't crumble (or explode - the common fuel mix is liquid hydrogen and liquid oxygen). The cool thing about staging is that you basically multiply the mass ratios of the different stages - so to get at a 100+ mass ratio, you could use three stages, each with a mass ratio of 5, for example. The drawback is that you end up littering your spent stages all over the place (their price is pretty much thrown away, and spaceship stages aren't exactly cheap), and you still need to use huge amounts of fuel to deliver a bit of payload.
Which is where the delta-V tricks come in. One great trick is using the target's atmosphere for the final braking - this is used all the time by craft returning to Earth, for example. Instead of having to nullify your whole orbital speed, you only need to shave off about 100 m/s or so to get you low enough into the atmosphere to get enough drag to "steal" the remaining orbital velocity. Doing the same with interplanetary velocities is a fair bit trickier, though - you only have a limited time to get rid of enough velocity to get you in a reasonable orbit. And the faster you need to brake, the stronger your spaceship structure must be - and stronger structure means more mass. Yet another reason to keep the ship/probe as tiny as possible.
Another great trick is using the gravity of other planets to modify your velocity - this needs a lot of planning, but allows you to both speed up and slow down without expending any fuel (other than the bit required for path corrections). This was used to great effect with the Voyager missions of the 70s - Voyager 1 has achieved a velocity of over 17 km/s thanks to its numerous gravity assists. Even then, this is only about half of Earth's orbital velocity - so you really want to make sure to steal as much velocity from Earth as possible on launch. The tricky part about this? For one, it's kind of slow. The path you take is likely going to be much longer than a direct path, especially if you need multiple gravity assists from multiple planets. The Voyager missions were possible mainly because in the 70s, the outer planets were aligned in a way that allowed the Voyagers to use Jupiter and Saturn (+ Uranus for Voyager 2) in a sequence of assists that increased their velocity "for free". The trick there? Those alignments don't happen all that often. Another alignment that would allow the same kind of mission happens about once in 170 years.
Now, the planet position issue isn't that much of a problem when travelling from Earth to Mars - for one, you don't need nearly as much delta-V as the Voyagers, and second, both Earth and Mars have much shorter orbits. But still, if we keep to modern-style rockets (as opposed to the so-called "Torchships", which would be capable of accelerating the whole trip, avoiding a lot of the issues mentioned here thanks to their massive exhaust velocities), position matters a lot. This gives rise to the familiar concept of launch windows (which are also used to great effect in drama - "if we don't go now, our next option is in 3 years, by which time we'd starve").
If you want to get somewhere on the lowest delta-V budget possible, Hohmann transfer orbits are your friend. However, they are also the slowest, and the most dependent on the alignment of the planets. Launch windows are quite periodic - again, the Voyager missions have launch windows every 175 years or so, and Mars missions around 780 days. The more delta-V you're willing to spend, the wider your launch window and the shorter your trip.
As an example, getting from Earth to Mars and back requires a minimum of about 30 km/s of delta-V. For comparison, the massive Saturn V rocket is only capable of about 18 km/s. It shouldn't be very surprising that we make the interplanetary probes as light as possible, and we don't bring them back again :) This trip would take about 17 months. That's quite long - it doesn't matter much for an unmanned probe, but any human crew would need to bring a lot of supplies, be pretty good at recycling and not get mad. Adding more delta-V to the budget allows us to trim this considerably - 53 km/s will allow you to do the trip in just two months. Of course, getting a delta-V of 53 km/s is well beyond our current capabilities. Ignoring the mass of the rather massive fuel tanks, you'd need a mass ratio of about 80 000. Yikes. Interestingly, increasing the budget to 94 km/s will only save you about half a month - adding more delta-V gives diminishing returns, since you're still relying heavily on using tricks - the more delta-V, the less effective these tricks are in comparison.
So, what about absolutely absurd, outrageous delta-Vs? The next obvious step is something that can accelerate the whole trip. We're already experimenting with ion drives for exactly this, but with the kind of acceleration we're getting, it's not really useful for humans. But let's imagine we have some fusion torch drive that gives us enough reachable delta-V. Pushing the delta-V to 370 km/s (remember how I said absurd delta-V?) makes the roundtrip about one month long. This corresponds to a constant acceleration of about 0.01 g. By this point, orbital mechanics are no longer much of a limitation, so adding more delta-V tends to give you close to a linear speedup - 0.1g acceleration requires about 1 100 km/s for a 12 day roundtrip, and 1g requires "only" 3 500 km/s for a 4 day roundtrip.
Obviously, once you get to a torchship, your original problem disappeared. Even for the 0.01g constant acceleration scenario, your velocity is huge compared to orbital velocities, so the cost of turning your ship around is relatively tiny (compared to going the whole way and back again), and the impact of all those manenuvering tricks are quite tiny as well (so you can shave off 1 km/s of my delta-V budget? Big deal...).
But as long as you're flying in a modern rocket, that takes 9 months to get to Mars... once you're on the interplanetary trajectory, there's no going back. Even if you expend all your remaining fuel, you're not even going to stop your ship, much less turn it around. You'll just have to sit out the rest of the trip :)
I assume that "halfway there" refers to being halfway along the transfer orbit from Earth to Mars, and that by "turn around" you mean reverse direction immediately. There is no reason a spacecraft "cannot" do this, but the reason it is entirely impractical is because of the propellant required to change the spacecraft trajectory.
Aborting an Earth-to-Mars transfer to return to Earth requires the spacecraft continue along its current interplanetary transfer orbit until it swings back to Earth -- but most importantly it requires that the transfer orbit be selected with a period so that the spacecraft encounters Earth. Without the appropriate timing, the spacecraft will return to where Earth was at launch, not where Earth will be when it reaches the same point. This type of abort scenario uses what is called a free-return trajectory, which was used during Apollo 13 (along with a subsequent propulsive manoeuvre to hasten the return and shift the splashdown location).
You can find a discussion of Earth-to-Mars abort scenarios in this paper -- see page 20. Both a free-return trajectory as well as a propulsive or gravity-assist return trajectory are discussed.
Here is a comparison of a 2-year free-return trajectory on the left and a propulsive abort trajectory on the right (from the above linked paper). Note the dotted green line on the right indicates the trajectory without any propulsive manoeuvre (which would not reach Earth at the correct timing).
There's no singular reason why a spacecraft would be unable to change its trajectory to return to Earth, it just requires the appropriate delta-v budget and time.
Depending on what the craft was initially designed for, it may not have the delta-v to execute such a course change.
For example, the delta-v remaining on a one-way Mars ship, even one that used a rocket-powered soft landing on Mars, would certainly not have the delta-v to return to Earth much sooner, if at all, compared to a slingshot.
By contrast, the delta-v remaining on a two-way Mars ship without a separate lander (from Earth, go to Mars, land, get back to orbit, return to Earth) could likely pull of the maneuver, though not necessarily in the most expedient way (dipping in closer to the sun than Earth's orbit to 'catch up' to it).
As a balancing point, a ship that was designed to go to Mars, stay in orbit while a lander dropped to the surface and returned, then take the next convenient transfer back to Earth is a toss up on whether or not it has the delta-v required to 'turn back'.
With all this in mind, the most time expedient return would be very expensive, delta-v wise, and the crew or supplies may run out of time waiting for a more fuel efficient transfer; especially if any refueling or resupplying was to be done at Mars.
This would be quite easy to try out yourself, just download the KSP trial and use a pre-built ship. The solar system isn't entirely the same, but you can see the relative merits of each maneuver with Duna, or download a mod to do it in a decent approximation of ours.
If you ask nicely enough in the forums, someone might record the answer for you in video form.
A short note on what delta-v is, in case you are not familiar: delta-v is a measure of the amount of change in velocity your craft can perform. If you are riding a bicycle, and going 4 miles per hour, and want to go 10 MPH, you need 6 MPH delta-v. You can provide this to your 'craft' on Earth's surface from energy expended against the friction of the surface due to gravity.
Because there is so little matter in the near-vacuum of space, there is hardly any friction with which to push off. The only (current) method of changing your velocity in space is by tossing mass in the opposite direction. It helps if you 'toss' the mass fast, which is why we use rocket fuel which burns and gets subsequently 'tossed' rather fast.
The other thing to note is that trajectories between objects in space are typically some variant of transfer orbits, not simply 'heading toward' something. Once an orbit is established, a maneuver is plotted that changes that orbit into one that will intercept another orbit at the time very close to when the target object will be there. "Turning around" then becomes not simply reversing course or 'pulling a 180', but again altering the orbit for a return transfer orbit that intersects the original body.
A very common one is the Hohmann Transfer Orbit, and the wiki article is a good place to start.
I'll try a less technical answer, because most people are unfamiliar with the orders of magnitudes involved in space travel, and sci-fi action movies taught us that flying in space is just like a ship cruising on the sea.
To escape the gravity of Earth, you need a lot of speed. You will need over 40000 km/h, this is more than 40 times faster than a jet airliner, and more than 10 times faster than the fastest fighter jet has ever flown. To reach this speed, you need a huge rocket engine and an insane amount of fuel.
In space, if you don't use gravity assist but want to rely on your own engines to turn back, you have to expend (almost) the same amount of energy to stop what you used to speed up at the beginning, and then spend that energy again to achieve the same speed in the other direction. So why not just carry three times as much fuel? Because unlike a car where you can just put a small barrel of extra fuel in your trunk, most of the weight of a spacecraft is made up by the fuel.
When you look at a picture of a spacecraft before launch, you'll see that most of it is fuel:
See that tiny little trapezoid near the top? That is the actual spaceship, all the rest is mostly fuel. So we'll just build our rocket 3 times larger, for the eventuality we want to turn back, right? Wrong! The humongous rocket on the picture was used to speed up that tiny little spacecraft at the top of it! To lift up all the extra fuel (which is heavier than the original entire rocket), you will need an even bigger rocket. And to lift off that even bigger rocket, you will need a much bigger rocket, and so on ...
There is an interesting article on xkcd, describing what you will need to return one of our small probes which is on the way to leave the solar system: it would require a rocket at least 60 times bigger than the one we used to send it up!
Orbital mechanics is weird. When on Earth, turning around and heading home is usually a good solution when something goes wrong. This isn't the case in space because of some strange quirks.
Stopping is hard
So with the first item, stopping is hard. This is because you can't build brakes in space. Which means to stop, you need a rocket just as big as the one that you used to get you going in the first place.
However, if you need to send a really big rocket, then you need an even bigger rocket to get that going in the first place.
We call this problem the "tyranny of the rocket equation".
This is why we almost always stop on planets where we can use brakes (or parachutes as you call them).
The shortest distance is curved
In general, earth is pretty flat. But if need to walk from a-b and there is a hill in the way, you would generally walk around the hill.
Space is the same. With orbital mechanics, there is a MASSIVE hill in the middle we call the sun.
Home is constantly moving
On the time scale of a trip to Mars, Earth will move. If you turned around and headed back again, it won't be there. It is possible that it is now "on the other side" of Mars, depending on the alignment of the stars.
As a rule, spacecraft will have enough fuel to accomplish it's intended mission path and some safety margin, but not more than that. Furthermore, since fuel is so huge part of the total weight, you will optimize the mission to take the most efficient suitable path, often relying various factors like choosing transit moment to fit a particular position of planets, using gravity assist and the like.
Furthermore, due to the way orbital mechanics work, if you're en route to Mars then the cheapest way (fuel-wise, not time-wise) to turn around is to go to Mars orbit and burn your engines there.
Even simply "stopping"(e.g. relative to sun) on the spot in middle of nowhere already requires more fuel than it took you to get up to speed, since the mission definitely was planned to use earth orbital speed and earth rotation speed to the advantage of the initial mission plan; and reversing also takes more fuel than expected since the earth is moving away from you in its orbit.
This means that if you have enough fuel for a particular trip to Mars and back, after you burn nearly half of it to start the initial trip, any significant changes will require more fuel - which you simply won't have.
