What is the required burn to keep a satellite at a Lagrangian point?

Question

When a satellite reaches a Lagrangian point, it has a non-zero velocity $v_1$ because of the transfer orbit in which it had already been. What burn, say, $\Delta v$, one needs if the satellite is about to be kept at that Lagrangian point? Given velocity $v_2$ after the burn applied to the spacecraft, shall we have $v_2 = 0$, i.e., $\Delta v = -v_1$?

Answer 1

What is the required burn to keep a satellite at a Lagrangian point?

tl;dr: typical station keeping delta-v for a halo orbit around Sun-Earth L1 or L2 points are of the order of 2 to 4 meters/sec per year based on a very old spacecraft (SOHO) and a future spacecraft (JWST).

---

I'll address the two closest and most used Lagrange points; L1 and L2. Generally spacecraft are put in halo or Lissajous orbits associated with (around) the points, not at the points themselves. This is done both for geometrical reasons (avoid the Sun in the line of sight for antenna-pointing or blockages) but there may be orbital mechanical reasons as well. See the extensive answers to Are large halo orbits around L₁'s and L₂'s preferred over small orbits for reasons other than geometry?

I'll add a quick note, Lagrange points and halo (and other) orbits are mathematical manifestations of mathematics. They exist in a certain theoretical situation called the Circular Restricted Three Body Problem or CR3BP (or CRTBP). These concepts work approximately in the real world where orbits are not circular and there are more than three bodies.

Whether you are at L1 or L2, or in a halo orbit around them, you are really orbiting around the larger body. If you are at or near the Sun - Earth L1 (e.g. DSCOVR, SOHO) you are really in an orbit around the Sun which is in resonance with the Earth. It would normally move around the Sun a little faster than Earth, but Earth pulls it back just enough to keep it orbiting with a period of one year.

There is a subclass of halo orbits around L1 and L2 in the CR3BP model that are actually stable, though the rest aren't. However in the real world due to there being other gravitational bodies around and the orbits not being circular, none of these are really stable.

According to this answer to ** the James Webb Space Telescope (JWST) only needs a station-keeping delta-v budget of 2 to 4 meters/sec per year to stay in its halo orbit around the Sun-Earth L2 point. It accomplishes this though careful planning and construction. JWST sits just in front of the most stable halo orbit so it would ten to wander toward the Earth, but this is balanced by solar photon pressure on its giant solar thermal shield, which tends to hold it there (roughly speaking). It will also execute very tiny delta-v maneuvers ever three weeks so that the errors are tiny and never allowed to grow.

I talk a lot about SOHO (Sun-Earth L1) station keeping in Is this what station keeping maneuvers look like, or just glitches in data? (SOHO via Horizons) and in the exciting report (if you like orbital mechanics) Roberts 2002 The SOHO Mission L1 Halo Orbit Recovery From the Attitude Control Anomalies of 1998 in Table 2 and the paragraph that follows, it says that SOHO originally needed about 2.4 meters/sec per year for station-keeping.

When a satellite reaches a Lagrangian point, it has a non-zero velocity 𝑣1 because of the transfer orbit in which it had already been.

Getting to halo orbits around L1 and L2 does not always require a classical "transfer orbit". You can sneak in with very little delta-v along what is called a "stable manifold" as SOHO did for example. It's not perfectly zero delta-v, but it is very low.

It is a lot like the opposite of what would happen if you started just Earthward of the halo orbit, you would drift out along the unstable manifold. Drifting in is like drifting out but backwards in time.

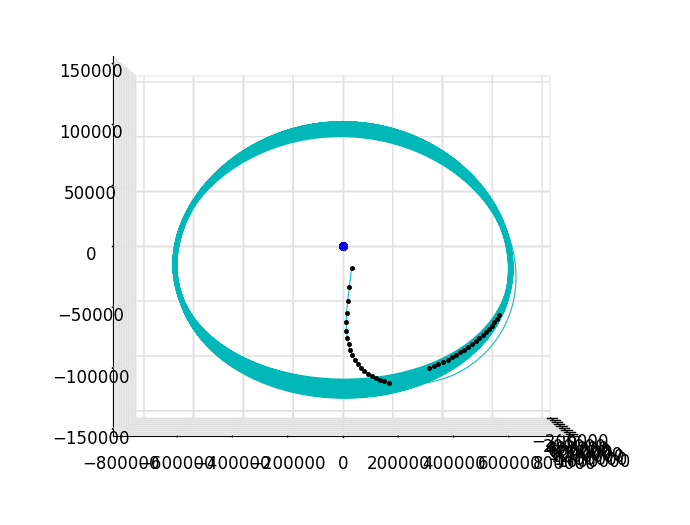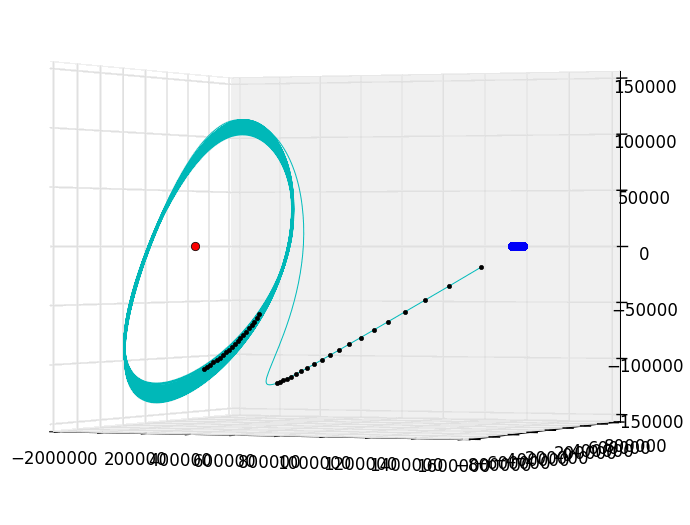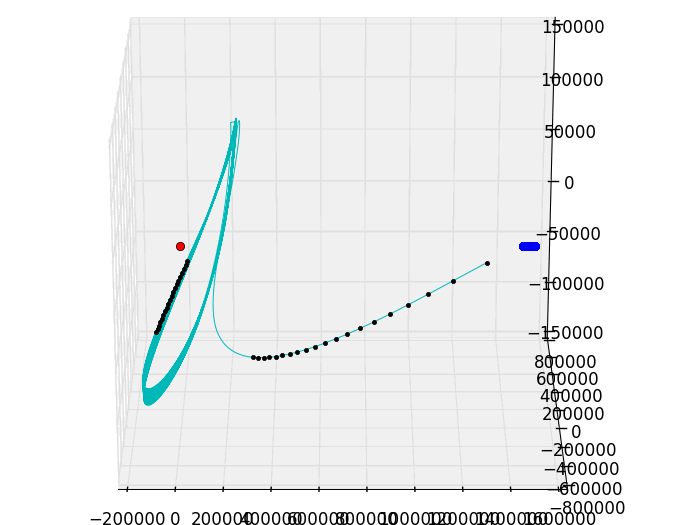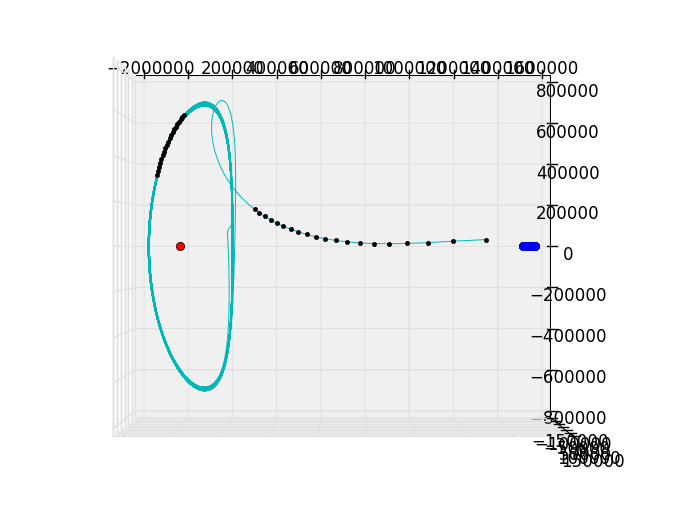
This is plotted data from Horizons from Is this what station keeping maneuvers look like, or just glitches in data? (SOHO via Horizons) using a script like this: https://pastebin.com/7XULFDea written when I was just starting to learn Python. Still images combined into a GIF using ImageJ. Data from https://ssd.jpl.nasa.gov/horizons small black dots are 1 day intervals, red dot is Sun-Earth L1 and blue blob represents the various places Earth is relative to L1.

Answer 2

For those Lagrangian points which are unstable, L1, L2 and L3, there is no equilibrium, and any movement off the point will accelerate further away, towards the Sun or Earth. For them, you would need to counteract v1 (and any gravitational forces undergone along the way) in order to reach a rest velocity with respect to the Lagrangian point plus you would have to use thrusters to remain there.

For stable Lagrangian points, L4 and L5, you would need to simply meet the required orbit parameters. There will still be a burn to match the orbit.

Answer 3

The Wind spacecraft has been orbiting the Earth-Sun L1 point since roughly May 2004. Originally it was put into a Lissajous orbit but was recently moved into a halo orbit in late 2020.

Typically, Wind uses ~0.13-0.20 kg of fuel per maneuver and four maneuvers per year (it had ~36 kg remaining as of December 16, 2021, i.e., ~45-60 years of fuel left). For Wind, ~0.14 kg of fuel corresponds to ~0.276 m/s of delta-v (changes slightly over time as its mass decreases with use of fuel, obviously). It is an ecliptic spinner (i.e., the spin axis is pointed toward the south ecliptic pole) and has a banded antenna about its midsection (i.e., like a ring around the center of the cylinder of the spacecraft bus) so it doesn't need to do extra maneuvers to reorient the spacecraf for communication with Earth. In contrast, the ACE performs maneuvers every other week or so. The difference is that ACE is a sun-pointed spinner (i.e., its spin axis is nearly directed at the sun). ACE needs to point in a specific direction for communication with Earth and because one of its plasma instruments is failing (so they canted the spin axis a little to rely more heavily on the less damaged anodes). This results in it using a lot more fuel per year than Wind.

In short, the fuel usage depends on the spacecraft.