How could a satellite follow Earth around the Sun while staying outside of Earth's orbit?

Question

I'm wondering if its possible for a satellite to follow Earth around the Sun while staying outside of its orbit. I wondering for the sake of space based solar infrastructure or just something constantly obstructing sunlight getting to earth.

I read something about objects in L4 or L5 zone taking about a year to orbit earth. I assume that might produce a similar result for what I'm looking for, and maybe its simpler? I'm having a hard time finding information about man made objects orbiting the sun, mostly because of mars trajectories articles.

Answer 1

If I understand the question as it is evolving, you are looking for an orbit that produces a solar eclipse; a complete shadow of the Sun on a small area of the Earth, and further that the object casting the shadow not be in an orbit around the Earth as in the question Is a sun-blocking orbit possible? but instead be in a heliocentric orbit.

That would mean that your object needs to produce an umbra at the Earth's surface, not just a penumbra.

Sun-Earth L1

Other answers have already pointed out that an object in a heliocentric orbit near Sun-Earth L1 would satisfy the orbital conditions:

Source

Remaining at Sun-Earth L1

In order to stay near Sun-Earth L1, the spacecraft would have to perform some station-keeping propulsive maneuvers using thrust. That could come from a rocket engine or ion engine or from a solar sail or some kind of electromagnetic sail producing thrust from the charged particles from the solar wind.

But how big would it have to be to produce an umbra on Earth?

Sun-Earth L1 is about 1.5 million kilometers from Earth. That is 1% of the distance from the Earth to the Sun. So the object would have to be 1% of the Sun's diameter to produce an umbra from SE L1. The Sun is about 1.4 million kilometers in diameter, so your object would have to be 1% of that or about 14,000 kilometers wide in order to cast a complete shadow of the Sun on Earth.

That's pretty big!

---

Source

Source: TimeAndDate.com's Umbra, Penumbra, and Antumbra: Why Are There 3 Shadows?

Answer 2

The only stable points that orbit at the same speed as Earth are the L4 and L5 points, as you mention, but there are some unstable ones as well. See this pic from NASA:

L4 and L5 remain ahead of and behind the Earth, whereas L1, L2 and L3 are inherently unstable. From your question, I'd suggest L4 and L5 would be best suited, unless you really need proximity to Earth, in which case L2 could suit your needs.

Answer 3

Note: the question has been radically re-written since this answer was written. Consequently, it is no longer relevant to the question.

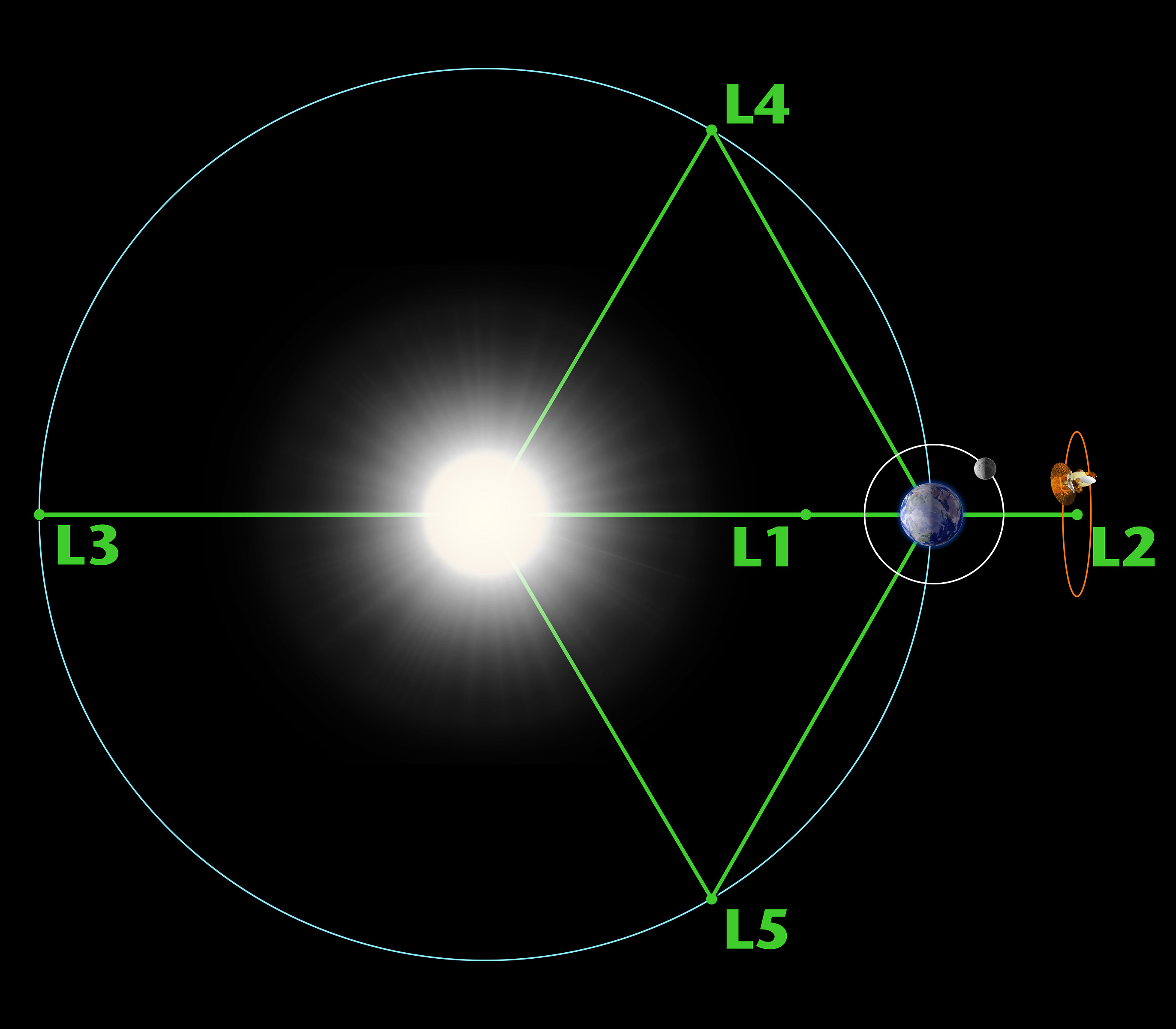
If you want an object to stay between the Sun and Earth, it has to be at the Earth-Sun L1 point, which is about 1.5 million km away. We already have stuff there: https://en.wikipedia.org/wiki/Solar_and_Heliospheric_Observatory

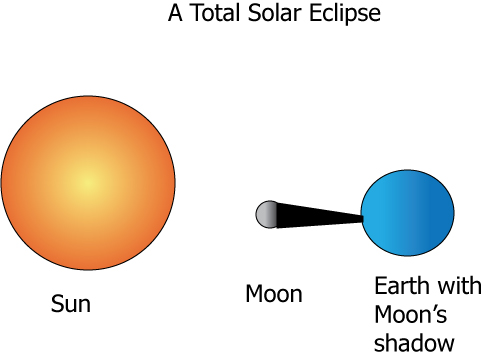
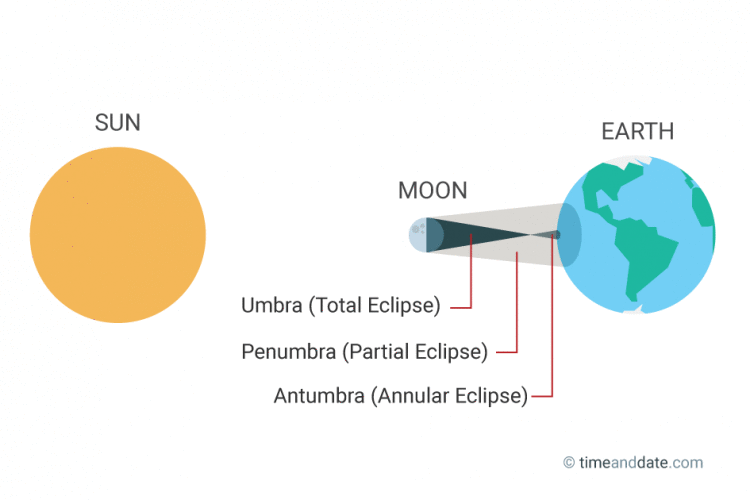
At L1, you can arrange for your object to cross the Sun from the perspective of any point on Earth. However, this is different than casting a shadow on Earth. In order to cast a shadow, you'll need your object to have at least the same angular size as the Sun, as seem from Earth. Conveniently, the Moon has approximately the same angular size as the Sun, and it's only 384,400 km away. In order to have to be able to eclipse the sun from L1, your object will need to be substantially larger than the Moon.

Now that we're talking about something enormous, the physics of Lagrange points comes into play. They're only stable(-ish) for objects of negligible mass compared to the primary and secondary. SOHO has negligible mass compared to the Earth and the Sun. A sun-shade several times the diameter of the Moon probably doesn't. You could try making it a disc rather than a sphere (to keep its mass down), but I expect that tidal forces would spin it out of alignment quite quickly. Its mass will also noticeably affect Earth's orbit, changing the position of the Earth-Sun L1. You'll end up with a complicated gravitational dance as both Earth and your object try to share a similar orbit. Possible outcomes include:

• The object collides with Earth.
• The object collides with the Moon
• The Moon gets tugged into a much more elliptical orbit, resulting in far higher tides that make a giant mess on Earth.
• The Moon gets ejected from Earth orbit, possibly colliding with Earth.
• The object gets ejected into an elliptical orbit around the Sun, where it probably eventually collides with Earth, Venus, or Mars. Meanwhile, Earth ends up in a somewhat lower orbit and more elliptical orbit, throwing another big wrench into the climate.
• Some combination of the above catastrophes, which may involve the object being a temporary second moon for a while.

Answer 4

You're on the right track looking up Lagrangian points, orbits where a small object can stay in the same relationship with two celestial bodies, one orbiting another. The one you are describing is the earth-sun L2 point, a point outside of earth's orbit around the sun. This Wikipedia page will tell you more.

Answer 5

With engines!

Orbiting at L1 is completely feasible, as long as your satellite regularly uses little bursts from its engines to keep it there. L1 is "unstable", meaning that a satellite without engines will eventually drift away from L1. But the closer your satellite stays to L1, the less fuel it requires to stay in place. Low thrust, high specific impulse engines such as ion thrusters are often used to keep satellites in the right orbit.