How do you calculate the ∆v required to increase apoapsis to a certain height?

Question

To calculate the ∆v needed to increase our apoapsis from a low orbit of 100km to 400km, we find the velocity at 100km using the semi major axis (which is 250km, in this case), and then find the difference between our current velocity, and the velocity at periapsis after the burn, giving the ∆v.

What if we aren't in an orbit, but on the ground. We want to increase our apoapsis to 100km by burning straight up (no gravity turn), but we can't use the orbital velocity formula, as we are burning radially, and not horizontally. We also don't know our semi major axis, as we don't know our periapsis. How would you go about calculating the ∆v required to increase apoapsis to 100km from the ground?

Answer 1

I’m not a space engineer, but I found this thread and I think it can answer your question. Delta V as a Function of Altitude

The lift-off delta v to a $100km$ altitude is in the range of about $1.4km/s$ for an ideal system.

And the answer with explained equation :

To just reach a height of 150km at least once, you don't need to achieve a true orbit (one where perigee misses the Earth). The size of your orbit depends on the specific energy of the orbit:

$ \epsilon=\frac{GM}{-2a} $

where a is the semi-major axis, G the universal gravitational constant, M the mass of the Earth, and epsilon the specific energy. You could multiply the universal gravitational constant by the mass of the Earth once and use it as a constant. In fact, it is a constant and has a name, the geocentric gravitational constant, and is equal to $398600 {km^3}/{sec^2}$ (assuming you don't want to convert your altitudes, radii, and semi-major axis to meters).

The semi-major axis is half the long axis. You need the long axis to be at least 150 km above the surface of the Earth (with the Earth's radius being 6378 km). So your a needs to be about 3265 km.

Your total energy is the sum of kinetic energy and potential energy. Your potential energy depends on your distance from the center of the Earth:

$ U=−\frac{GM}{r} $

where U is potential energy (per unit of mass) and r is your distance from the center of the Earth. At the surface of the Earth, r is equal to the Earth's radius.

The difference between your total specific energy and your potential energy has to equal your kinetic energy, which is:

$ T=\frac{1}{2}{v^2} $

where T is your kinetic energy (per unit of mass) and v is your speed. $1.7 km/sec$ should get you to an altitude of 150 km.

You can combine all of the above into one equation and only have to determine the proper semi-major axis to make it work:

$ v=\sqrt{GM\frac{2}{r}−\frac{1}{a}} $

Your r will always equal the Earth's radius, while a will be affected by what altitude you want to achieve.

Now mind you, your 'orbit' will virtually hit the center of the Earth, so you'll only achieve the desired altitude once. If you want to actually orbit at some given altitude, your semi-major axis needs to big enough for perigee to miss the Earth, as well.

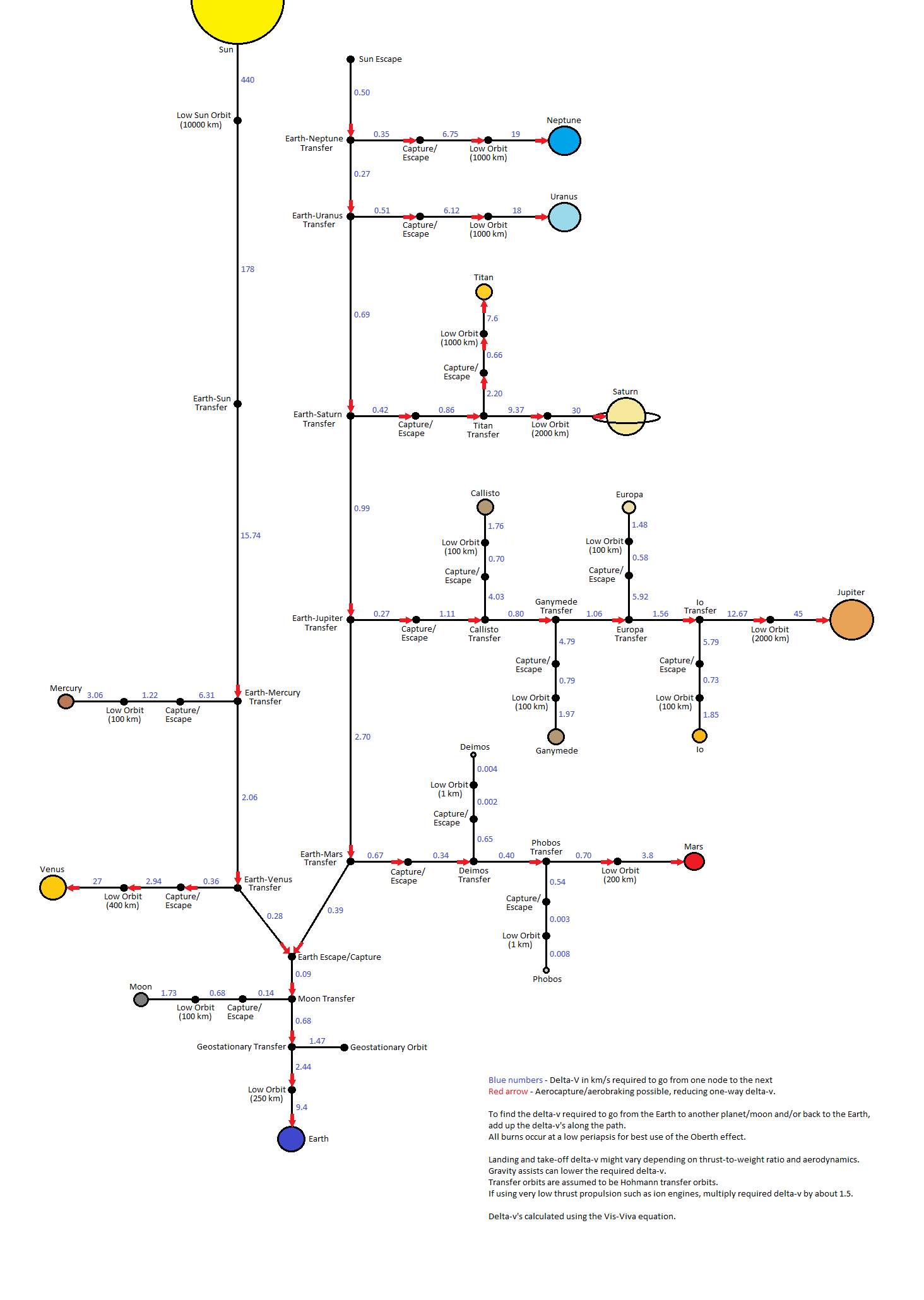
Bonus: the Delta-V Map of the Solar System