Is a ballistic Jovian capture using the Galilean moons possible from interplanetary entry?

Question

While spacecraft like Galileo and Juno did use gravity assist to supplement their insertion burns, as answered here, is it possible to enter Jupiter's orbit without a capture burn?

Answer 1

Yes, but really no.

Preamble:

I built a little 2D trajectory simulation (2D because the Galilean moons are more or less coplanar) to test out trajectories (Jupiter + Galilean moons gravity, RK4, 10s time step). My original intention was to perform an exhaustive search across 4 dimensions of initial conditions but this was prohibitively expensive (computationally) at sufficient resolution. Luckily, I stumbled upon a few representative cases (sets of initial conditions) that help answer the question.

Technicality: Parabolic + 1

A completely valid interpretation of "from interplanetary entry" is anything with $V_{\infty}>0$, or 'parabolic +1' infinitesimal speed unit. In this interpretation it is not too difficult to find a trajectory that captures around Jupiter ($V_{\infty} < 0$), here is one using a Callisto flyby ($1.14$ $R_{Callisto}$ close approach):

(Jupiter to scale, moons horribly not to scale; they're tiny!)

Though $V_{\infty} < 0$ indicates an elliptical orbit in a Keplerian orbit scenario, sufficiently large orbits (in the real, n-body world) grey the region of applicability of the Keplerian approximation. In this example the captured orbit is huge, significantly larger than Comet Shoemaker–Levy 9's orbit:

• Semi-major axis: ~600 $R_{Jupiter}$ or ~0.28 AU (!)
• Eccentricity: 0.9904
• Apoapsis: ~1200 $R_{Jupiter}$ or ~0.55 AU (!)
• Period: ~13 years (greater than a Jovian year!)

Reality: things of interest (typically) come from Earth

For a direct, Earth to Jupiter interplanetary trajectory the Jupiter arrival $V_{\infty}$ will of course be higher than 0. Borrowing from work on my answer to Orbital Mechanics and Launching into the Sun, the minimum Jupiter arrival $V_{\infty}$ (for a Oct'21 - Oct'22 launch) was 5.73 km/s (there are probably more creative trajectories that could lower that value but it is at the very least representative, Edit: using HORIZONS data Juno's $V_{\infty}$ was 5.3 km/s and Galileo's was 5.6 km/s).

This trajectory uses Ganymede & Callisto flybys (very close; $1.04$ $R_{Ganymede}$ and $1.13$ $R_{Callisto}$ respectively!) but is unable to capture:

Also note that the change in $V_{\infty}$ is significantly less than in the above 'parabolic +1' case, despite flying by the much more massive Ganymede and Callisto:

This presents a sort of reverse-Oberth tyranny of gravity assists where because only the direction changes ($V_{\infty,start}=V_{\infty,end}$ in the moon frame), and the direction change is inversely proportional to $V_{\infty}$, a faster flyby has a smaller effect.

This is also why Callisto sees such prominence here because it is a) the second most massive Galilean moon (after Ganymede), and b) the furthest Galilean moon from Jupiter. This means that the $V_{\infty}$, in Callisto's eyes, is the lowest of the Galilean moons for a given interplanetary arrival trajectory.

---

Edit: In response to comment:

A 'direct prograde encounter' with Calisto should then remove speed relative to Jupiter though, not add, and vice versa for a direct retrograde encounter

Here is a (roughly) prograde encounter with Callisto ($1.41$ $R_{Callisto}$):

which definitely adds Jupiter relative (inertial in this coordinate frame) velocity. Shown a different way:

where the red dot is the starting point of the trajectory.

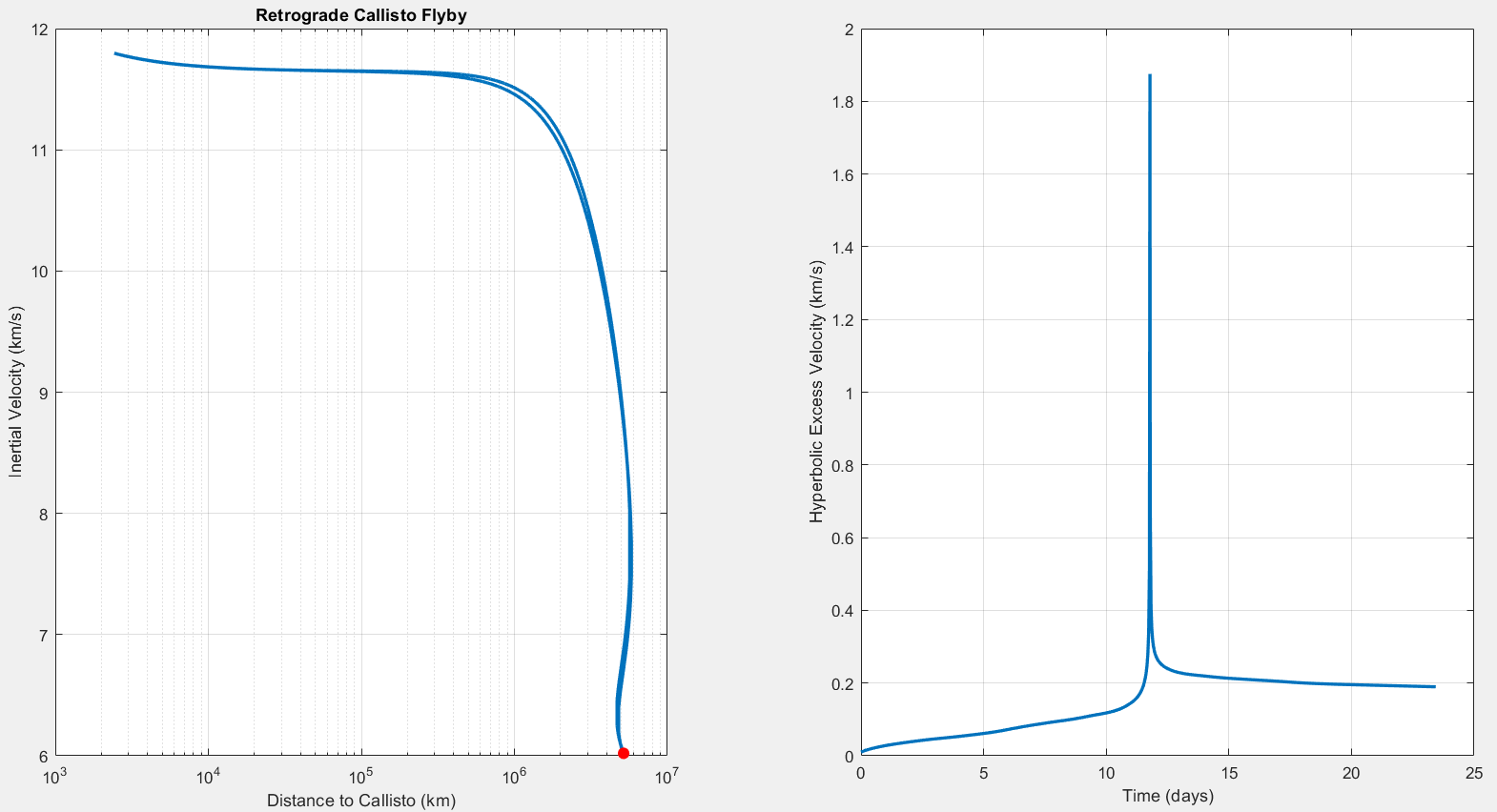
And for a retrograde encounter ($1.01$ $R_{Callisto}$):

Though showing a gain in velocity, this is an artifact of the numerical integration used and is not realized in the below plots:

Answer 2

Edit: re-reading the question this is not an answer, which is looking for solutions involving gravity assists only while this only covers aerobraking orbit adjustment

---

The study here (summary is section 6.4) found that for an example mission profile while substantial mass/cost savings would be possible it would involve several years of orbital shaping to step orbit inward, the radiation environment incredibly hostile (and applying over that multi year period) notably Juno has stayed out of the region of peak radiation due to radiation concerns by using a polar orbit, and still required careful design to survive. Assuming aim of aerobraking is to reach the moons passing through the peak radiation regions becomes necessary.

The biggest concern is that while the gas pressures are very low at the proposed pass altitude the very high velocity produces temperatures around 39,000 kelvin. The low pressure means this temperature will not necessarily melt the bulk of the craft in a single pass but will attack any low mass exposed components like antenna or foil thermal covering, requiring more complex design and allowance for erosion.

Moving to a higher pass altitude lowers the pressure/heat load but does not do much for the speed, so still problematic and massively increases the number of needed passes going from years to decades to reach final orbit.

This tends to suggest that aerobraking at Jupiter is not automatically a more efficient choice than carrying a rocket engine and fuel, unless for other reasons the craft is radiation shielded and physically robust.