Are there uses for these 'quasi iso-propic' supersynchronous transfer orbits?

Question

In my answer to What are the benefits of supersynchronous transfer orbits? I stumbled upon an interesting outcome when considering the total $\Delta V$ cost from an inclined low altitude parking orbit to geostationary orbit using a supersynchronous transfer orbit. The maneuver profile is detailed below, requiring 3 burns:

• supersynchronous transfer orbit injection burn:
  • perigee @ low parking orbit height
  • apogee > geosynchronous height (> 35786 km, supersynchronous)
  • no change in inclination
  • $\Delta V_1 = \sqrt{\mu (\frac{2}{r_{park}}-\frac{1}{\frac{r_{park}+r_{Apo}}{2}})} - \sqrt{\frac{\mu}{r_{park}}}$
• combined plane change + perigee raising burn @ transfer orbit apogee:
  • raise perigee to synchronous height (35786 km)
  • change inclination to 0°
  • $V_{Apo1} = \sqrt{\mu (\frac{2}{r_{Apo}}-\frac{1}{\frac{r_{park}+r_{Apo}}{2}})}, V_{Apo2} = \sqrt{\mu (\frac{2}{r_{Apo}}-\frac{1}{\frac{r_{sync}+r_{Apo}}{2}})}$
  • $\Delta V_2 = \sqrt{V_{Apo1}^2 + V_{Apo2}^2 - 2V_{Apo1} \cdot V_{Apo2}\cos{i}}$ (cosine law)
• circularize @ perigee:
  • $\Delta V_3 = \sqrt{\mu (\frac{2}{r_{sync}}-\frac{1}{\frac{r_{sync}+r_{Apo}}{2}})} - \sqrt{\frac{\mu}{r_{sync}}}$

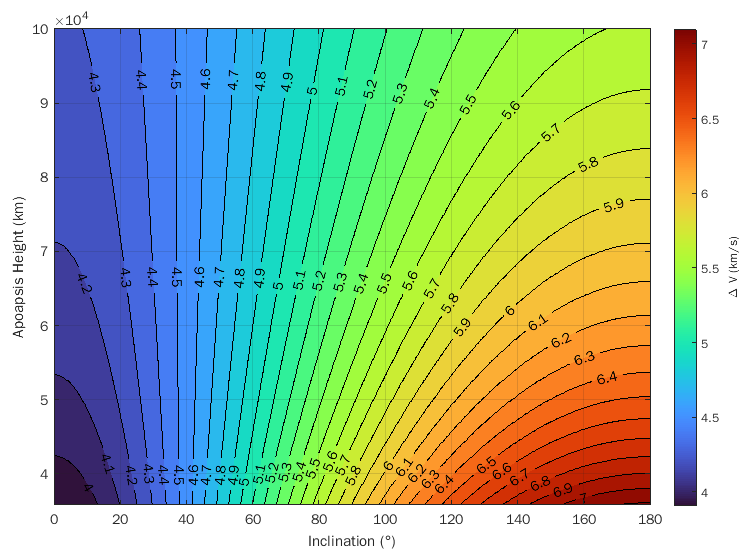
The total $\Delta V$ is the sum of all 3 burns (though typically the satellite is on its own for the final 2). Plotting the total $\Delta V$ over a wide range of inclinations and transfer orbit apogees yields this (250 km parking orbit assumed):

Around ~40° it looks like the contours go vertical, indicating a sort of iso-propic line (if you will) where the total $\Delta V$ cost is independent of transfer orbit apogee (!wow). But, note that the $\Delta V$ required of the satellite (final 2 burns) decreases with increased transfer orbit apogee.

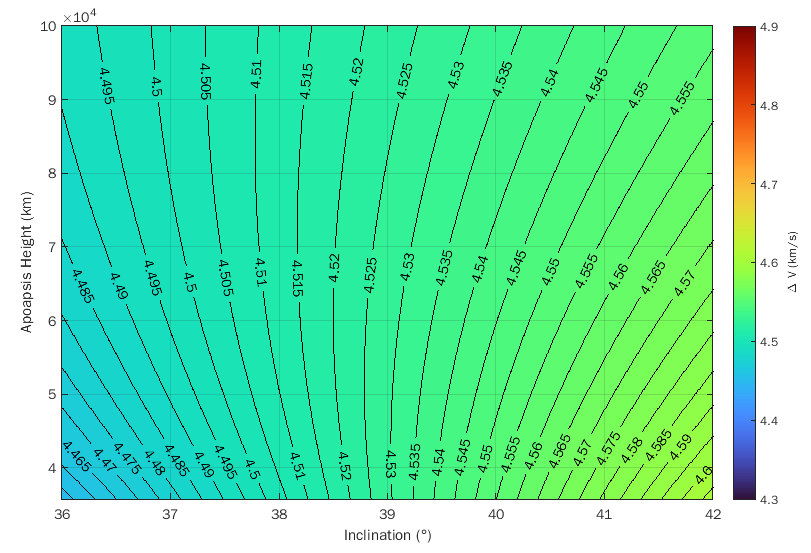
Upon closer inspection there is no proper vertical line/invariant inclination, but these 5 m/s contours show that there is a region of quasi iso-propicness around 39° inclination (5 m/s in the scheme of 4+ km/s seems pretty invariant to me):

There is no benefit for a traditional upper stage + payload combination because while the total $\Delta V$ stays the same, the distribution between launcher and payload changes by as much as ~20% for the apogee scale shown in the plots.

Consider though, a single spacecraft responsible for all of the (admittedly high) $\Delta V$. Only one transfer orbit apogee can be used per mission, of course. However, is there any reason why sitting in a ~39° inclined low Earth orbit with the ability to target geostationary orbit from any transfer orbit apogee would make sense? Even if it does make sense, are there more practical solutions?

---

Re: Comments, here are the 3 burns individually. The first and last don't deal with inclination and thus only depend on transfer orbit apogee (click to enlarge):

Answer 1

What you are seeing here is the result of the inefficiencies in the orbital transfer strategy employed, in the transition region between direct transfers and bi-elliptic transfers.

When transferring between two circular orbits with some inclination, eventually the "degenerate" bi-elliptic transfer (with infinite apoapsis) will become optimal, provided the target orbital radius or the inclination is large enough.

At that point, it's a flat transfer cost, independent of inclination change:

$$\Delta v = \left(\sqrt{2} - 1\right)\left(\sqrt{\frac{2\mu}{r_1}} + \sqrt{\frac{2\mu}{r_2}}\right)$$

The other strategy, or course, is to do a direct arc, splitting the inclination change between the two burns (not evenly!).

At ~40°, targeting synchronous orbits, you are in the transition region between these two, costs are about the same.

But they have the opposite reaction to increased altitude!

• High-apopasis bi-elliptic transfers become cheaper when the target orbit becomes higher (after some inclination-dependent threshold)
• Direct arc transfers become more expensive when the target orbit becomes higher.

You're using a hybrid approach, which has two theoretical inefficiencies:

1. It has a limited transfer apoapsis. (bi-elliptic inefficiency)
2. It does not put any of the inclination change in the first burn. (direct arc inefficiency)

With a hybrid approach like that, the altitude-dependent delta-v cost trends will cancel eachother out for some inclination-altitude region, and that's what you have.

Are there uses for these 'quasi iso-propic' supersynchronous transfer orbits?

I do not think so. The only reason to do large inclination changes, like ~40°, is to reach synchronous orbits from launch sites not on the equator. But that's only one useful altitude, so the rest of the quasi iso-propic line isn't used.