6
$\begingroup$

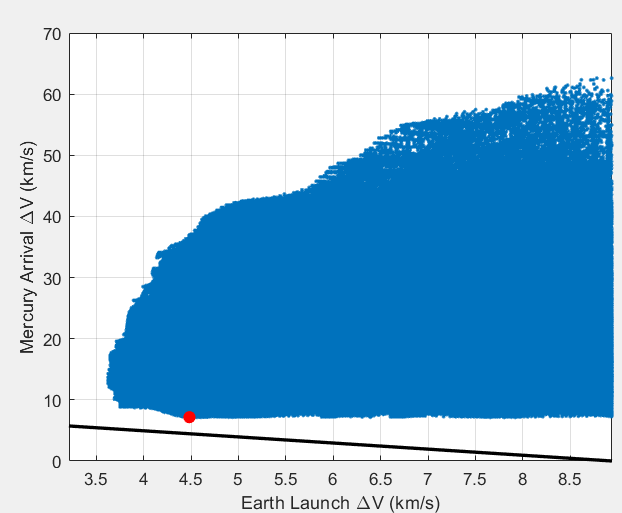
This question may be a crucial follow-up to this one because in this answer it is calculated that the payload to Mercury without a gravity assist would probably be minimal, so if already a moderate reduction in the spacecrafts speed could be reached , that would cause a relative big change in payload for the better.
From this answer I've learned that Starship just isn't designed to survive the heat it would absorb by traveling so close to the Sun. But let's assume it would have extra temperature-resistant layers or heat shields for radiative cooling, while it already has a heat shield on its belly for aerobraking.

Since the purpose of the Venus gravity assist would be to slow the spacecraft down, and Starship would have the necessary heat shields, aerobraking in the upper atmosphere of Venus would be an extra option, although the effect of it would probably be hard to calculate.

Finally, couldn't it be advantegeous if Starship could catch up slowly with Mercury somewhere between its perihelion and aphelion, where the planets orbital speed would increase from almost 39 km/s to 59 km/s in just 44 days ?
Or is it around aphelion, because then there's more time to adjust the speed ?

Improve this question
$\endgroup$
7
  • 2
    $\begingroup$ Starship's heat shield is not a sun shield. It is made to allow aerodynamic braking at hypersonic speeds in an atmosphere for a few minutes, not to withstand intense radiant heating in vacuum for months on end. The two things would be designed in completely different ways, and a sun shield would largely rule out aerobraking as an option. And the biggest problem for reaching Mercury is simple delta-v. The problem isn't lack of time, it's that you physically can't carry the propellant required to get the job done with chemical propulsion without multiple expendable stages. $\endgroup$
    – Christopher James Huff
    Commented Jan 17, 2022 at 15:41
  • 2
    $\begingroup$ Starship has potential to be the first really general purpose spacecraft, able to reach much of the solar system via orbital propellant transfer and ISRU, but I don't think you fully understand how extreme a case Mercury is. You might conceivably be able to reach it with Starship, but you'd be far better off with a specialized spacecraft designed from the ground up for that mission, with multiple, large expendable stages or some completely different propulsion technology like nuclear or solar-thermal rockets, and likely an orbit-only component if you intend anything to come back. $\endgroup$
    – Christopher James Huff
    Commented Jan 17, 2022 at 15:49
  • 1
    $\begingroup$ You're not protecting against near-Mercury levels of insolation with some backside coating that can survive a pass through an atmosphere at Venus-flyby velocities. I don't know why you're even trying to do this with radiative cooling on the sun-facing surface. What you need is something much closer to MESSENGER's sunshade, and more insulation on the actual vehicle to protect against Mercury itself. And no, the remaining delta-v is not the escape velocity of Mercury...that's the minimum it would be if Mercury was alone in otherwise-empty space. In reality it's deep in the Sun's gravity well. $\endgroup$
    – Christopher James Huff
    Commented Jan 17, 2022 at 20:52
  • 2
    $\begingroup$ For a thorough telling of MESSENGER's trip to Mercury, there is this paper $\endgroup$
    – BrendanLuke15
    Commented Jan 17, 2022 at 21:35
  • 1
    $\begingroup$ @BrendanLuke15 Yes, that's very thorough, I wouldn't dare to ask for an answer that detailed ! :) $\endgroup$
    – Cornelis
    Commented Jan 18, 2022 at 9:21

2 Answers 2

Reset to default
7
$\begingroup$

A single Venus flyby helps, but it does not make the journey possible for Starship.

Below is a plot of 2025-2030 trajectories from Earth to Mercury that flyby Venus once. They are plotted by the Earth departure $\Delta V$ (X-axis, from 250 km LEO) and Mercury arrival $\Delta V$ (Y-axis, Mercury relative speed @ 0 km altitude):

possible trajectories

There were over 1.7 million possible trajectories (blue dots). The minimum total $\Delta V$ is 11.65 km/s and is shown as the red dot. The black line shows the $\Delta V$ limit of a payload-less Starship, 8.94 km/s. A trajectory only works if it lies below this line.

More elaborate, multi flyby trajectories are increasingly more expensive (computationally) to look for and are beyond my skillset. Looking at McAdams, J et al. "MESSENGER - Six primary maneuvers, six planetary flybys, and 6.6 years to Mercury orbit," Advances in the Astronautical Sciences. 142. (2012)., a definitive telling of MESSENGER's journey to Mercurian orbit, we can sum up the major maneuvers to find the $\Delta V$ cost for a multi flyby comparison:

Maneuver: $\Delta V$ (km/s) Notes
"Launch" (from LEO) 3.94 back calculated from a C3 of $16.4$ $km^2/s^2$ & 250 km LEO, Figure 5
DSM-1 0.3156 Table 5
DSM-2 0.2274 Table 5
DSM-3 0.0722 Table 5
DSM-4 0.2467 Table 5
DSM-5 0.1778 Table 5
MOI 0.8617 Table 5
DeOrbit for Landing 0.02 cost to change 15265 km x 207 km orbit to 15265 km x 0 km
Landing 3.98 velocity at periapsis in 15265 km x 0 km orbit
MESSENGER Total 5.84 (1.90 by spacecraft)
Total for Landing 9.84 sum of all maneuvers

Even if Starship took the hyper-efficient (but 6.6. years long!) MESSENGER trajectory it is still unable to land on Mercury.

It is probably also safe to say that the MESSENGER like trajectory is at the upper limits of efficiency, likely representing a minimum $\Delta V$ budget for getting to, and by extension landing on, Mercury.

Improve this answer
$\endgroup$
7
  • 1
    $\begingroup$ Impressive work ! I will need some time to check it, as far as it's possible for me. $\endgroup$
    – Cornelis
    Commented Jan 21, 2022 at 9:13
  • 1
    $\begingroup$ @Cornelis total for MESSENGER is from LEO for an equal comparison with Starship, I will make that clearer (launch probably isn't the right word!) $\endgroup$
    – BrendanLuke15
    Commented Jan 21, 2022 at 12:20
  • 1
    $\begingroup$ @Cornelis no. For verifying & quantifying the possible gravity assists from Venus I used a Lambert's problem solver to create two 2D matrices containing departure and arrival $v_{\infty}$ values for each 'leg' of the proposed trajectory. In my answer Leg 1 is Earth to Venus and Leg 2 is Venus to Mercury for a total of four 2D matrices. However, two of these matrices contain the same metric, the $v_{\infty}$ at Venus.... $\endgroup$
    – BrendanLuke15
    Commented Jan 21, 2022 at 14:39
  • 1
    $\begingroup$ @Cornelis ... If I search in those two matrices for a date when the $v_{\infty}$ are equal then a hyperbolic flyby of Venus could be possible for that set of 3 dates (the chosen Venus flyby date that matches $v_{\infty}$ at Venus for leg 1 & leg 2 will determine the Earth departure & Mercury arrival dates). Most Lambert solvers will give you a $v_{\infty}$ vector (x,y,z components)..... $\endgroup$
    – BrendanLuke15
    Commented Jan 21, 2022 at 14:47
  • 1
    $\begingroup$ @Cornelis .... You can use the Venus inbound (leg 1) & outbound (leg 2) $v_{\infty}$ vectors to determine the hyperbolic deflection angle ($2\nu$ in Wikipedia) for the flyby. Using that Wikipedia equation you can then determine the $r_p$ value necessary for the gravity assist flyby to work. Obviously if the $r_p$ is below the planet's surface or in the atmosphere then the gravity assist flyby is not possible. $\endgroup$
    – BrendanLuke15
    Commented Jan 21, 2022 at 14:52
5
$\begingroup$

One thing to keep in mind is the trade off between use of gravity assists, the alignment of the planets at launch and the mission duration.

It is theoretically possible to use multiple passes of the Earth, the Moon, Venus and Mercury to provide gravity assists in a variety of permutations. The permutations increase further still if modest deltaV changes can be applied at any point in the mission.

BepiColombo is using one Earth, two Venus and six Mercury gravity assists and will take 7 years. Even for a single gravity assist from Venus there may be a considerable time delay in transit between planets. The option may not be available in some (or possibly most or many years) and each option is likely to provide a different level of benefit depending on how optimal the alignment is and other factors.

https://en.wikipedia.org/wiki/Gravity_assist#Limits

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.