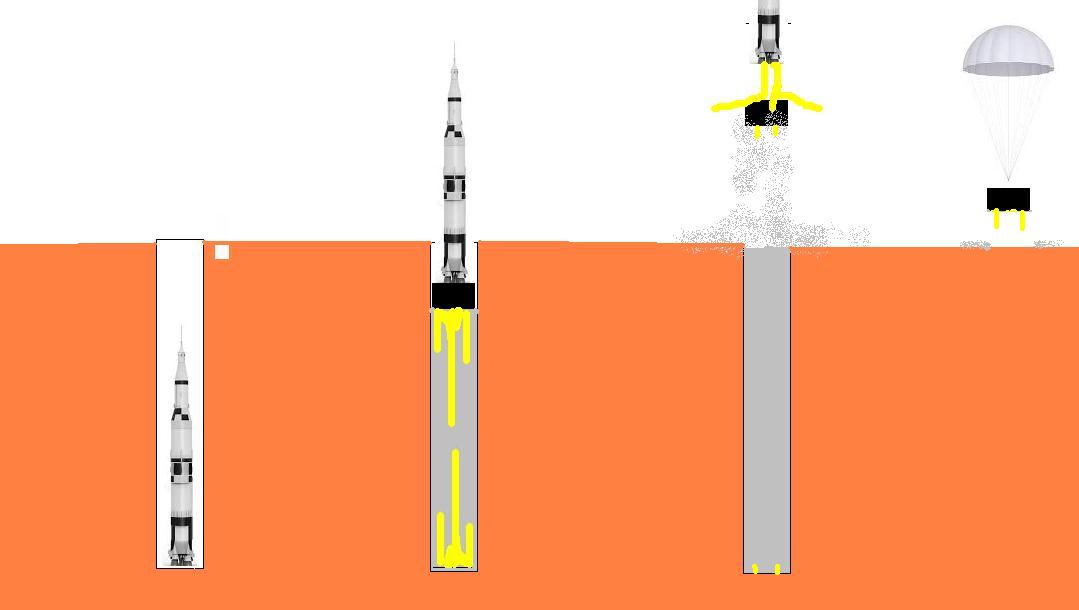
Benefits:
- less fuel needed to reach orbit
Problems:
- To get enough speed to matter, you need a long track = a deep shaft. 3 km is about the practical limit.
- To get the rocket (let's say this weighs 550 tons, the starting weight of a Falcon 9) to a usable speed in only 3 km of track, you need lots of power. The F9 uses 9 Merlin engines to accelerate off the pad, so the amount of power you need: Falcon 9 FT: 7,607 kN (1,710,000 lbf) thrust at liftoff.
The RR Trent 800 jet engine is available as an aircraft engine or as a powerplant. It produces 36 MW of shaft power or 80 klbf/360 kN of thrust. Using that conversion as a shortcut, you need 21 of these engines to provide as much power as the F9 at liftoff, or 760 MW to lift the rocket with its platform at the same rate of acceleration as an F9 coming off the pad. Linear motors capable of handling 760 MW are going to be huge (count on a few hundred tons), not to mention the power lines needed to carry that much power. 760 MW is equivalent to 30 TGV trains.
You have to brake the platform from halfway up the shaft to avoid it shooting out (at a few hundred tons, there's no way it can make a soft landing). That halves the speed you can reach.
you lose the ability to start the engines and test them before launch. Any malfunction when you start the engines will lead to a crash and loss of mission. You can't start the engines while you're still in the tunnel. You don't want the rocket exhaust blasting up the sides of the rocket.
If you want to move the piston via compressed gas, how much gas do you need?
- let's assume 550 tons of rocket plus 50 tons of piston
- piston diameter 5 m, area 19.6 m3
- shaft 1 km deep (borrowing from Russell's answer)
- pressure needed to lift this off the ground: 600,000/19600 = 30 kg/dm2 (30 bar)
- amount of gas needed to fill a shaft 1 km deep to 30 bar: specific weight of air is 1.2 g/m3, shaft contains 19600 m3 x 30 bar is 705 tons of air.
So if you want to do this, you need 705 tons of rocket exhaust at 30 bar to fill the shaft.
That's more than the F9 first stage uses for its entire burn, so instead of saving fuel you're using more. You've also traded making the rocket 10% smaller (a negligible saving, you're just saving one barrel section of aluminum by shortening the tanks a bit) for having to build and maintain a shaft 1 km deep with a gas-tight seal between the piston and the shaft.
And this calculation ignores some problems:
- When you use a rocket to generate this gas, the gas cools as it leaves the rocket engine, lowering its pressure.
- Rocket engines become inefficient when they have to run against a high exhaust pressure.
- I've ignored that the gas needs to accelerate the piston and rocket, the pressure I calculated just holds the piston off the ground without accelerating it.
So both electric and pneumatic power for moving the piston are not feasible.
 https://metro.co.uk/2018/03/30/russia-tests-4000mph-hypersonic-missile-near-impossible-to-shoot-down-7428694/
https://metro.co.uk/2018/03/30/russia-tests-4000mph-hypersonic-missile-near-impossible-to-shoot-down-7428694/