2
$\begingroup$

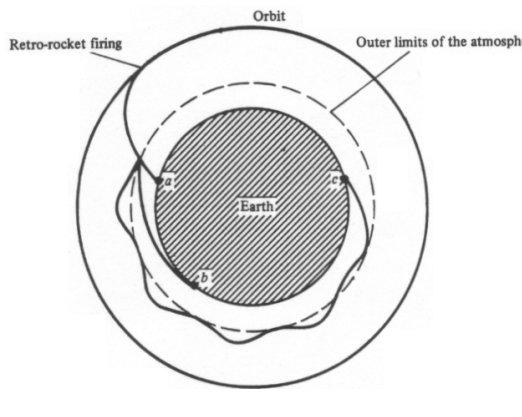
While descending from orbit can the air be thin enough for a glider type blimp to slowly shed speed?

Could a blimp skim the atmosphere around a planet, slowly decreasing air speed, without the need of a traditional heat shield?

Like a hydroplaning ship on water, the glider-like blimp would be using the air under it to skip most upper atmosphere during orbital entry. I don't think this has been attempted yet, or does this have a name other than 'skip reentry'?

https://www.newscientist.com/article/dn10288-inflatable-cushions-to-act-as-spacecraft-heat-shields/

It would look like the picture below, but with more skips at smaller angles of attack, and more orbits.

enter image description here

enter image description here

Improve this question
$\endgroup$
3

1 Answer 1

Reset to default
6
$\begingroup$

Let's begin by the last question: a company named JP Aerospace proposed an "double" airship that could reach LEO using electric propulsion. See this previous question.

Concerning reentry, the problem is to do the aerobreaking fast enough so your vehicle can withstand the heat generated and aerodynamic forces of the atmosphere at the given altitude. Perhaps in a planet that have a very thin atmosphere this could be possible, but on Earth you would need retro rockets to decelerate to safe velocity levels.

This happens because the atmosphere's density has an exponential behavior so when you are gradually slowing using just the drag, you will fall in a high drag zone very fast (even with the buoyancy, since the density is still very low for considerable force).

The orbital decay by atmospheric drag usually follows a profile as illustrated in the image:

enter image description here

Improve this answer
$\endgroup$
1
  • 1
    $\begingroup$ Could you plot the height loss (aka the time derivative w.r.t. height) of those curves? Else it is a bit hard to assess how much height you loose in how much time. A few km/day should still be safe, no? Also could you expand your answer on which differential equation you solved to generated the curves and which eccentricity you used. $\endgroup$
    – AtmosphericPrisonEscape
    Commented Nov 23, 2016 at 0:51

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.