I recreated your scenario using Wolfe's Spreadsheet. Here's a screen capture:
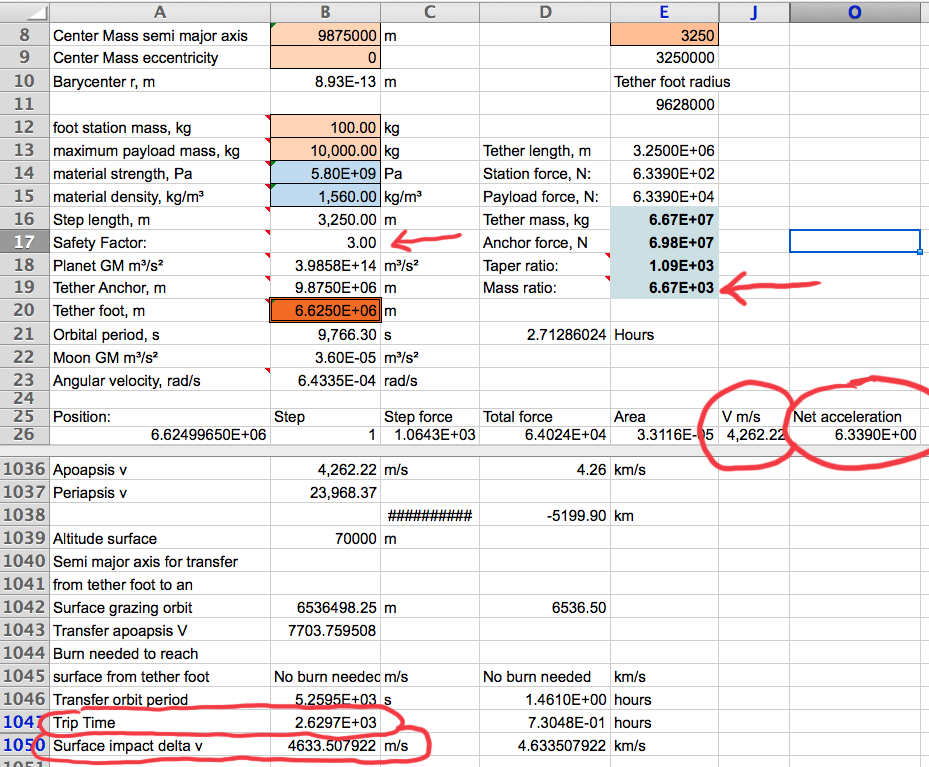
The numbers you cited match mine fairly closely. A very nice tool Hohmann fan has made available. I intend to link to his page from several of my tether blog posts.
The bottom of the spreadsheet answers some of the your questions. I called 70 kilometers the altitude where the craft starts serious aerobraking. Which is just a guess, to be honest.
The ship would be moving 4.6 km/s at that altitude. It would 7.3e-1 hours to reach this altitude which comes out to 43 minutes.
Scratch that - it is a mistake. Thank you for calling that to my attention, uhoh. The bottom of the spreadsheet was a transfer orbit from a 250 km apogee to a 70 km perigee. But in this scenario perigee is well below earth's surface and the ship re-enters well before perigee.
Here is a pic of Kim's scenario: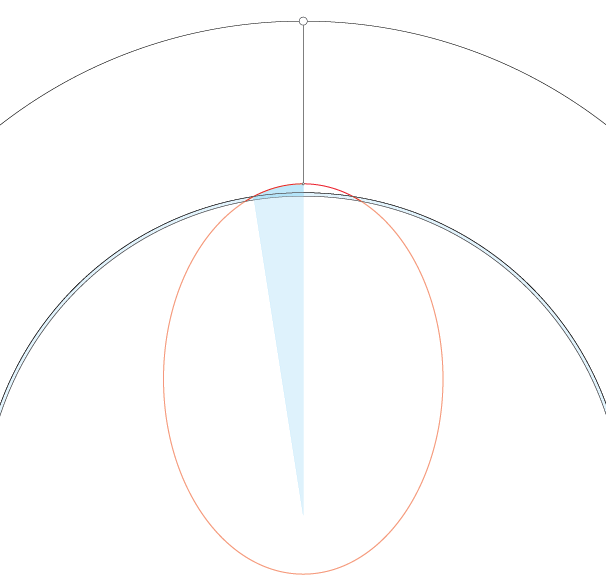
The eccentricity of the suborbital ellipse is .698. Semi major axis of that ellipse is about 3902 kilometers. Given that semi major axis, the period would be 2425 seconds.
But only a fraction of that period is swept out from release at apogee to atmospheric entry at 70 km. That fraction of the ellipse is shaded blue.
To get area of the fraction of an ellipse swept out, Kepler scaled the ellipse to make it a circle. Which is what I did. I also scaled radius of our circle to 1 to eliminate some arithmetic.
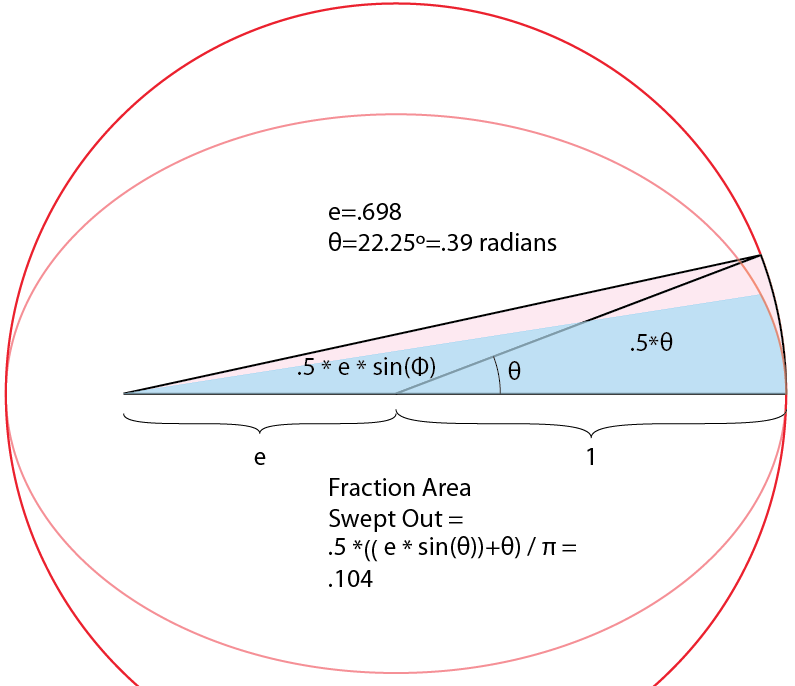
To get area swept out, Kepler would add area of a triangle and a wedge. Which is what I did above. In this case a little more than 1/10 of the ellipse.
.104*2425 seconds is about 252 seconds. Or about 4 minutes and 12 seconds.
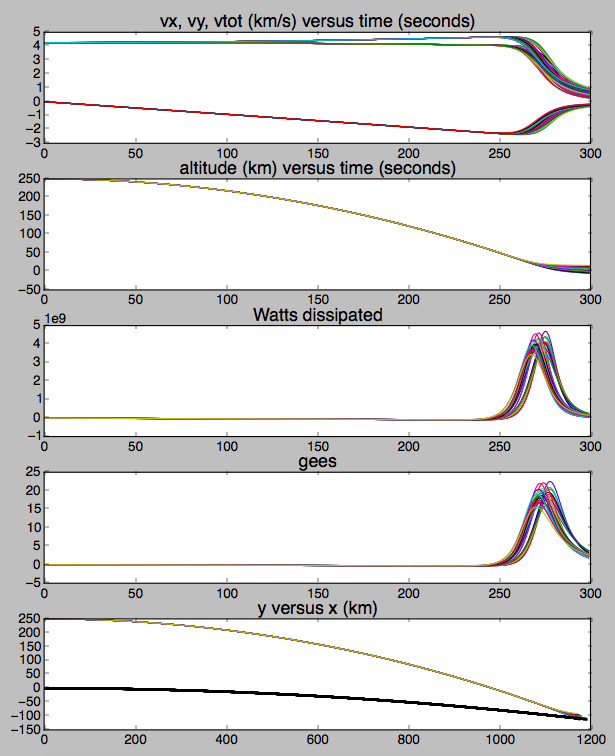
After release from a 250 km altitude, the spacecraft would start serious aerobraking in about 4 minutes.
At 70 km altitude I get a flight path angle of 19.2º. Horizontal velocity would be be about 4.38 km/s. Vertical velocity would be 1.53 km/s.
The short path from a 70 kilometer altitude to earth's surface doesn't give much time to shed the 4.64 km/s velocity.
As the capsule descends gravity will increase slightly, centrifugal acceleration decreases rapidly while deceleration from dynamic pressure increases rapidly.
I whomped up a first order Runge Kutta model of this drop from the tether foot at a 250 km altitude to earth's surface. A few different scenarios:
Dropping in a Vacuum
Time to Impact:
279 seconds
Impact Velocity: 4.63 km/s.
This is within about 4% of what I got using Kepler's method to get time of flight and the Vis Viva equation to get speed at 6378 km (earth's radius).
Dropping into earth's atmosphere
To get atmosphere density at an altitude I use $exp(-altitude/scale height)*1.225 kg/m^3$. I call 8500 meters the scale height. Dynamic pressure is $.5*\rho*v^2$). Force newtons is dynamic pressure x drag coefficient x cross sectional area capsule.
Scenario 1: 6500 kg, 1.85 meter radius, drag coefficient .5
Time to impact: 354 seconds
Impact velocity: 150 meters/sec which is about 333 mph.
Max Q: 217 kilo pascals.
Max aerobraking acceleration: 18 g's
I've been told 90 kilo pascals has been a Max Q for descent. I am guessing 217 kilo pascals is unacceptable.
Scenario 2: 6500 kg, 2.9 meter radius, drag coefficient .5
Time to impact: 416 seconds
Impact velocity: 92 meters/sec which is about 205 mph.
Max Q: 87 kilo pascals.
Max aerobraking acceleration: 18 g's
Here Max Q is less than 90 kilo pascals. This may be acceptable.
I don't know what how to figure heating.
If made of Zylon with a safety factor of 3, the lower tether would have a taper ratio of about 1090. Tether mass to payload ratio would about about 6,670.
I think with that great length it would have a large enough cross sectional ratio that impacts would be inevitable in the LEO volume which has lots of debris and satellites. As much as I love vertical tethers, I don't believe they could survive close to earth.
A rotovator might be a way to catch suborbital flights as well as release re entering craft into the atmosphere at suborbital speeds. John Carmack has already tweeted to Musk if he can land a booster on an ocean platform, he should be able to rendezvous with a rotovator. Musk agreed, sort of.