6
$\begingroup$

Following the links in this comment by @Uwe I've found some interesting sources of information on Ham (amateur) radio operators trying to listen in on Apollo transmissions. One of them is Sven Grahn's Tracking Apollo-17 from Florida.

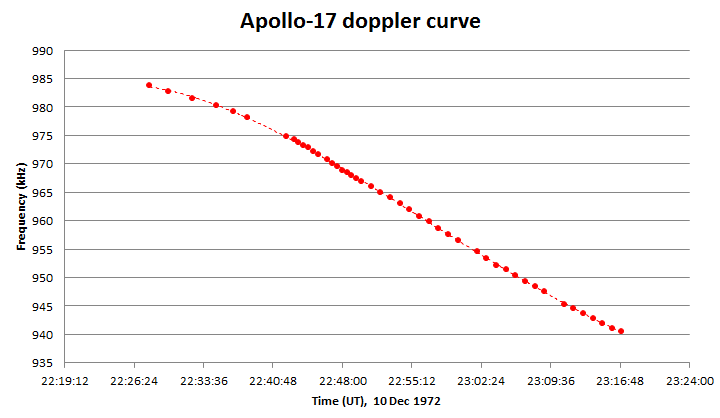
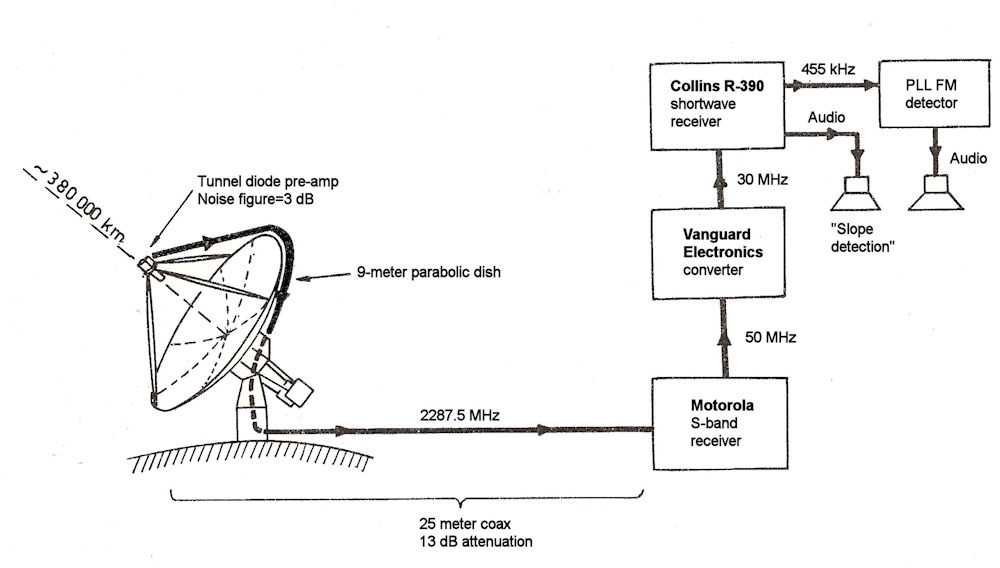
Ham's setting up a 9 meter dish to receive signals from the Moon, and a doppler shift measurement (offset) of the received signal at around 2287.5 MHz as the spacecraft orbited the near side of the Moon. From Tracking Apollo-17 from Florida.

It looks like there will be at least 50 kHz of doppler shift for one orbit of the near hemisphere of the moon, eyeballing the graph.

50 kHz divided by 2287.5 MHz is about 22.9 ppm. Multiply that by the speed of light and I get a velocity change of 6557 m/s. Using a GM_moon of 4.905E+12 m^3/s^2 and an altitude of 60km, I esitmate the orbital speed of only 1650 m/s.

That makes the estimate of the change in line-of-sight velocity four times larger than the orbital velocity. I can understand twice, since it changes from coming towards to going away. But not four times.

Question: What is the explanation for such a large doppler shift?

Images from Sven Grahn's Tracking Apollo-17 from Florida

enter image description here

enter image description here

enter image description here

Improve this question
$\endgroup$
2
  • $\begingroup$ Sven Grahn needed a very stable reference oszillator to measure a doppler shift of only 23 ppm. Propbably at least an oven xtal oscillator holding the xtal at constant temperature. But I remember a report about a Ham (amateur) radio operator using an xtal oscillator buried into the ground about 1 or 2 meters to keep the crystal at very constant temperature. Of course the oscillator should be buried some days before to stabilize the temperature. $\endgroup$
    – Uwe
    Commented Dec 23, 2017 at 21:28
  • $\begingroup$ 13 dB attenuation for 25 m coax or 52 dB for 100 m, that is pretty much. Nowadays the are cables with only 5,5 dB per 25 m or 22 dB per 100 m at 2.3 GHz. But flexible waveguides would be better at higher price. $\endgroup$
    – Uwe
    Commented Dec 23, 2017 at 22:17

1 Answer 1

Reset to default
5
$\begingroup$

From your link:

If the orbit had been perfectly circular at the 128.2 minute period the doppler shift for a simple transmitter would have been = 2287.5 x 1000 x 1.58/300000= ± 12 kHz. For a coherent transponder the doppler shift would be almost double this number (doppler shift on both uplink and downlink), i.e. 46 kHz.

Improve this answer
$\endgroup$
3
  • $\begingroup$ If Apollo 8 was moving towards the Earth the shift is +12 kHz, when moving away -12 kHz, the difference is 24 kHz and the double of that is 48 kHz. $\endgroup$
    – Uwe
    Commented Dec 23, 2017 at 19:48
  • 1
    $\begingroup$ OK I see that now, thanks! Also this sounds vaguely familliar. The Apollo downlink system was constantly receiving an uplink signal from earth and generating a downlink frequency using a stable offset. This provides NASA with a continuous monitor of their doppler shift without having to build a separate system. The period of 128 minutes still seems long for that altitude, but I'll look into that later. $\endgroup$
    – uhoh
    Commented Dec 24, 2017 at 3:31
  • 4
    $\begingroup$ @uhoh: that method is very old, the german V2 of WW II used this doppler shift method for engine shut down at the right velocity. A signal was send from ground to the rocket, the frequency was doubled and send back to ground. The ground station compared both frequencies and measured the doppler shift. When the rference value was reached, a shut down command was send from ground to the rocket. Precise measurement of doppler shift without the need for two very stable atomic clocks at ground and in the rocket. $\endgroup$
    – Uwe
    Commented Dec 24, 2017 at 10:22

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.